Published online by Cambridge University Press: 11 February 2015
In this paper we extend Bourgain’s double recurrence result to the Wiener–Wintner averages. Let 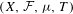 $(X,{\mathcal{F}},{\it\mu},T)$ be a standard ergodic system. We will show that for any
$(X,{\mathcal{F}},{\it\mu},T)$ be a standard ergodic system. We will show that for any 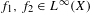 $f_{1},f_{2}\in L^{\infty }(X)$, the double recurrence Wiener–Wintner average
$f_{1},f_{2}\in L^{\infty }(X)$, the double recurrence Wiener–Wintner average 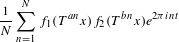 $$\begin{eqnarray}\frac{1}{N}\mathop{\sum }_{n=1}^{N}f_{1}(T^{an}x)f_{2}(T^{bn}x)e^{2{\it\pi}int}\end{eqnarray}$$
$$\begin{eqnarray}\frac{1}{N}\mathop{\sum }_{n=1}^{N}f_{1}(T^{an}x)f_{2}(T^{bn}x)e^{2{\it\pi}int}\end{eqnarray}$$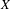 $X$ independent of
$X$ independent of  $t$ as
$t$ as 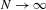 $N\rightarrow \infty$. Furthermore, we will show a uniform Wiener–Wintner double recurrence result: if either
$N\rightarrow \infty$. Furthermore, we will show a uniform Wiener–Wintner double recurrence result: if either 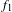 $f_{1}$ or
$f_{1}$ or 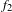 $f_{2}$ belongs to the orthogonal complement of the Conze–Lesigne factor, then there exists a set of full measure such that the supremum on
$f_{2}$ belongs to the orthogonal complement of the Conze–Lesigne factor, then there exists a set of full measure such that the supremum on  $t$ of the absolute value of the averages above converges to
$t$ of the absolute value of the averages above converges to  $0$.
$0$.