Article contents
On non-contractible periodic orbits for surface homeomorphisms
Published online by Cambridge University Press: 19 March 2015
Abstract
In this work we study homeomorphisms of closed orientable surfaces homotopic to the identity, focusing on the existence of non-contractible periodic orbits. We show that, if  $g$ is such a homeomorphism, and if
$g$ is such a homeomorphism, and if  ${\hat{g}}$ is its lift to the universal covering of
${\hat{g}}$ is its lift to the universal covering of  $S$ that commutes with the deck transformations, then one of the following three conditions must be satisfied: (1) the set of fixed points for
$S$ that commutes with the deck transformations, then one of the following three conditions must be satisfied: (1) the set of fixed points for 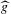 ${\hat{g}}$ projects to a closed subset
${\hat{g}}$ projects to a closed subset  $F$ which contains an essential continuum; (2)
$F$ which contains an essential continuum; (2)  $g$ has non-contractible periodic points of every sufficiently large period; or (3) there exists a uniform bound
$g$ has non-contractible periodic points of every sufficiently large period; or (3) there exists a uniform bound 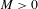 $M>0$ such that, if
$M>0$ such that, if  $\hat{x}$ projects to a contractible periodic point, then the
$\hat{x}$ projects to a contractible periodic point, then the 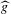 ${\hat{g}}$ orbit of
${\hat{g}}$ orbit of 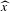 $\hat{x}$ has diameter less than or equal to
$\hat{x}$ has diameter less than or equal to  $M$ . Some consequences for homeomorphisms of surfaces whose rotation set is a singleton are derived.
$M$ . Some consequences for homeomorphisms of surfaces whose rotation set is a singleton are derived.
Information
- Type
- Research Article
- Information
- Copyright
- © Cambridge University Press, 2015
References
- 4
- Cited by

