No CrossRef data available.
Article contents
A new mean ergodic theorem for tori and recurrences
Published online by Cambridge University Press: 07 October 2019
Abstract
Let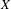 
$X$ be a finite-dimensional connected compact abelian group equipped with the normalized Haar measure 
$\unicode[STIX]{x1D707}$. We obtain the following mean ergodic theorem over ‘thin’ phase sets. Fix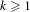 
$k\geq 1$ and, for every 
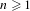$n\geq 1$, let 
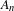$A_{n}$ be a subset of 
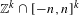$\mathbb{Z}^{k}\cap [-n,n]^{k}$. Assume that 
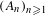$(A_{n})_{n\geq 1}$ has 
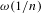$\unicode[STIX]{x1D714}(1/n)$ density in the sense that 
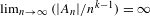$\lim _{n\rightarrow \infty }(|A_{n}|/n^{k-1})=\infty$. Let 
$T_{1},\ldots ,T_{k}$ be ergodic automorphisms of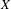 
$X$. We have
$$\begin{eqnarray}\frac{1}{|A_{n}|}\mathop{\sum }_{(n_{1},\ldots ,n_{k})\in A_{n}}f_{1}(T_{1}^{n_{1}}(x))\cdots f_{k}(T_{k}^{n_{k}}(x))\stackrel{L_{\unicode[STIX]{x1D707}}^{2}}{\longrightarrow }\int f_{1}\,d\unicode[STIX]{x1D707}\cdots \int f_{k}\,d\unicode[STIX]{x1D707},\end{eqnarray}$$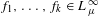
$f_{1},\ldots ,f_{k}\in L_{\unicode[STIX]{x1D707}}^{\infty }$. When the 
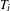$T_{i}$ are ergodic epimorphisms, the same conclusion holds under the further assumption that 
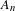$A_{n}$ is a subset of 
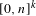$[0,n]^{k}$ for every 
$n$. The density assumption on the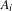 
$A_{i}$ is necessary. Immediate applications include certain Poincaré style recurrence results.MSC classification
- Type
- Original Article
- Information
- Copyright
- © Cambridge University Press, 2019
References
Bergelson, V. and Gorodnik, A.. Weakly mixing group actions: a brief survey and an example. Modern Dynamical Systems and Applications. Cambridge University Press, Cambridge, 2004, pp. 3–25.Google Scholar
Bergelson, V. and Gorodnik, A.. Ergodicity and mixing of noncommuting epimorphisms. Proc. Lond. Math. Soc. (3)
95 (2007), 329–359.CrossRefGoogle Scholar
Evertse, J.-H., Schlickewei, H. P. and Schmidt, W. M.. Linear equations in variables which lie in a multiplicative group. Ann. of Math. (2)
155 (2002), 807–836.CrossRefGoogle Scholar
Einsiedler, M. and Ward, T.. Ergodic Theory with a View Towards Number Theory
(Graduate Text in Mathematics, 259)
. Springer, London, 2011.Google Scholar
Furstenberg, H.. Recurrence in Ergodic Theory and Combinatorial Number Theory. Princeton University Press, Princeton, NJ, 1981.CrossRefGoogle Scholar
Ghioca, D. and Nguyen, K. D.. The orbit intersection problem for linear spaces and semiabelian varieties. Math. Res. Lett.
24 (2017), 1263–1283.CrossRefGoogle Scholar
Schlickewei, H. P..
S-unit equations over number fields. Invent. Math.
102 (1990), 95–107.CrossRefGoogle Scholar
Schlickewei, H. P.. Multiplicities of recurrence sequences. Acta Math.
176 (1996), 171–243.CrossRefGoogle Scholar
Schmidt, K. and Ward, T.. Mixing automorphisms of compact groups and a theorem of Schlickewei. Invent. Math.
111 (1993), 69–76.CrossRefGoogle Scholar
Schmidt, W.. The zero multiplicity of linear recurrence sequences. Acta Math.
182 (1999), 243–282.CrossRefGoogle Scholar
Schmidt, W. M.. Linear Recurrence Sequences, Diophantine Approximation (Cetraro, Italy, 2000)
(Lecture Notes in Mathematics, 1819)
. Springer, Berlin, 2003, pp. 171–247.Google Scholar