Published online by Cambridge University Press: 26 May 2020
Kifer, Peres, and Weiss proved in [A dimension gap for continued fractions with independent digits. Israel J. Math.124 (2001), 61–76] that there exists 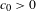 $c_{0}>0$ , such that
$c_{0}>0$ , such that 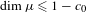 $\dim \unicode[STIX]{x1D707}\leq 1-c_{0}$ for any probability measure
$\dim \unicode[STIX]{x1D707}\leq 1-c_{0}$ for any probability measure  $\unicode[STIX]{x1D707}$ , which makes the digits of the continued fraction expansion independent and identically distributed random variables. In this paper we prove that amongst this class of measures, there exists one whose dimension is maximal. Our results also apply in the more general setting of countable branched systems.
$\unicode[STIX]{x1D707}$ , which makes the digits of the continued fraction expansion independent and identically distributed random variables. In this paper we prove that amongst this class of measures, there exists one whose dimension is maximal. Our results also apply in the more general setting of countable branched systems.