Published online by Cambridge University Press: 23 March 2021
This is the first of a series of two papers dealing with local limit theorems in relatively hyperbolic groups. In this first paper, we prove rough estimates for the Green function. Along the way, we introduce the notion of relative automaticity which will be useful in both papers and we show that relatively hyperbolic groups are relatively automatic. We also define the notion of spectral positive recurrence for random walks on relatively hyperbolic groups. We then use our estimates for the Green function to prove that 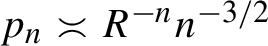 $p_n\asymp R^{-n}n^{-3/2}$ for spectrally positive-recurrent random walks, where
$p_n\asymp R^{-n}n^{-3/2}$ for spectrally positive-recurrent random walks, where 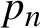 $p_n$ is the probability of going back to the origin at time n and where R is the inverse of the spectral radius of the random walk.
$p_n$ is the probability of going back to the origin at time n and where R is the inverse of the spectral radius of the random walk.