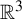 $\mathbb {R}^3$
$\mathbb {R}^3$Published online by Cambridge University Press: 18 October 2021
In this paper we focus on compacta 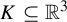 $K \subseteq \mathbb {R}^3$ which possess a neighbourhood basis that consists of nested solid tori
$K \subseteq \mathbb {R}^3$ which possess a neighbourhood basis that consists of nested solid tori  $T_i$. We call these sets toroidal. Making use of the classical notion of the geometric index of a curve inside a torus, we introduce the self-geometric index of a toroidal set K, which roughly captures how each torus
$T_i$. We call these sets toroidal. Making use of the classical notion of the geometric index of a curve inside a torus, we introduce the self-geometric index of a toroidal set K, which roughly captures how each torus 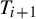 $T_{i+1}$ winds inside the previous
$T_{i+1}$ winds inside the previous 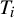 $T_i$ as
$T_i$ as  $i \rightarrow +\infty $. We then use this index to obtain some results about the realizability of toroidal sets as attractors for homeomorphisms of
$i \rightarrow +\infty $. We then use this index to obtain some results about the realizability of toroidal sets as attractors for homeomorphisms of 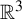 $\mathbb {R}^3$.
$\mathbb {R}^3$.