Article contents
Flattening functions on flowers
Published online by Cambridge University Press: 01 December 2007
Abstract
Let T be an orientation-preserving Lipschitz expanding map of the circle 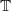 . A pre-image selector is a map
. A pre-image selector is a map  with finitely many discontinuities, each of which is a jump discontinuity, and such that τ(x)∈T−1(x) for all
with finitely many discontinuities, each of which is a jump discontinuity, and such that τ(x)∈T−1(x) for all 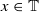 . The closure of the image of a pre-image selector is called a flower and a flower with p connected components is called a p-flower. We say that a real-valued Lipschitz function can be Lipschitz flattened on a flower whenever it is Lipschitz cohomologous to a constant on that flower. The space of Lipschitz functions which can be flattened on a given p-flower is shown to be of codimension p in the space of all Lipschitz functions, and the linear constraints determining this subspace are derived explicitly. If a Lipschitz function f has a maximizing measure S which is Sturmian (i.e. is carried by a 1-flower), it is shown that f can be Lipschitz flattened on some 1-flower carrying S.
. The closure of the image of a pre-image selector is called a flower and a flower with p connected components is called a p-flower. We say that a real-valued Lipschitz function can be Lipschitz flattened on a flower whenever it is Lipschitz cohomologous to a constant on that flower. The space of Lipschitz functions which can be flattened on a given p-flower is shown to be of codimension p in the space of all Lipschitz functions, and the linear constraints determining this subspace are derived explicitly. If a Lipschitz function f has a maximizing measure S which is Sturmian (i.e. is carried by a 1-flower), it is shown that f can be Lipschitz flattened on some 1-flower carrying S.
Information
- Type
- Research Article
- Information
- Copyright
- Copyright © Cambridge University Press 2007
References
- 1
- Cited by

