Published online by Cambridge University Press: 10 August 2018
The fact that the euclidean algorithm eventually terminates is pervasive in mathematics. In the language of continued fractions, it can be stated by saying that the orbits of rational points under the Gauss map 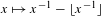 $x\mapsto x^{-1}-\lfloor x^{-1}\rfloor$ eventually reach zero. Analogues of this fact for Gauss maps defined over quadratic number fields have relevance in the theory of flows on translation surfaces, and have been established via powerful machinery, ultimately relying on the Veech dichotomy. In this paper, for each commensurability class of non-cocompact triangle groups of quadratic invariant trace field, we construct a Gauss map whose defining matrices generate a group in the class; we then provide a direct and self-contained proof of termination. As a byproduct, we provide a new proof of the fact that non-cocompact triangle groups of quadratic invariant trace field have the projective line over that field as the set of cross-ratios of cusps. Our proof is based on an analysis of the action of non-negative matrices with quadratic integer entries on the Weil height of points. As a consequence of the analysis, we show that long symbolic sequences in the alphabet of our maps can be effectively split into blocks of predetermined shape having the property that the height of points which obey the sequence and belong to the base field decreases strictly at each block end. Since the height cannot decrease infinitely, the termination property follows.
$x\mapsto x^{-1}-\lfloor x^{-1}\rfloor$ eventually reach zero. Analogues of this fact for Gauss maps defined over quadratic number fields have relevance in the theory of flows on translation surfaces, and have been established via powerful machinery, ultimately relying on the Veech dichotomy. In this paper, for each commensurability class of non-cocompact triangle groups of quadratic invariant trace field, we construct a Gauss map whose defining matrices generate a group in the class; we then provide a direct and self-contained proof of termination. As a byproduct, we provide a new proof of the fact that non-cocompact triangle groups of quadratic invariant trace field have the projective line over that field as the set of cross-ratios of cusps. Our proof is based on an analysis of the action of non-negative matrices with quadratic integer entries on the Weil height of points. As a consequence of the analysis, we show that long symbolic sequences in the alphabet of our maps can be effectively split into blocks of predetermined shape having the property that the height of points which obey the sequence and belong to the base field decreases strictly at each block end. Since the height cannot decrease infinitely, the termination property follows.