Article contents
An answer to Furstenberg’s problem on topological disjointness
Published online by Cambridge University Press: 10 April 2019
Abstract
In this paper we give an answer to Furstenberg’s problem on topological disjointness. Namely, we show that a transitive system 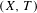 $(X,T)$ is disjoint from all minimal systems if and only if
$(X,T)$ is disjoint from all minimal systems if and only if 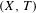 $(X,T)$ is weakly mixing and there is some countable dense subset
$(X,T)$ is weakly mixing and there is some countable dense subset 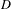 $D$ of
$D$ of 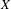 $X$ such that for any minimal system
$X$ such that for any minimal system  $(Y,S)$, any point
$(Y,S)$, any point 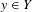 $y\in Y$ and any open neighbourhood
$y\in Y$ and any open neighbourhood  $V$ of
$V$ of  $y$, and for any non-empty open subset
$y$, and for any non-empty open subset 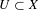 $U\subset X$, there is
$U\subset X$, there is 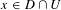 $x\in D\cap U$ such that
$x\in D\cap U$ such that 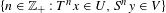 $\{n\in \mathbb{Z}_{+}:T^{n}x\in U,S^{n}y\in V\}$ is syndetic. Some characterization for the general case is also given. By way of application we show that if a transitive system
$\{n\in \mathbb{Z}_{+}:T^{n}x\in U,S^{n}y\in V\}$ is syndetic. Some characterization for the general case is also given. By way of application we show that if a transitive system 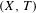 $(X,T)$ is disjoint from all minimal systems, then so are
$(X,T)$ is disjoint from all minimal systems, then so are  $(X^{n},T^{(n)})$ and
$(X^{n},T^{(n)})$ and 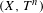 $(X,T^{n})$ for any
$(X,T^{n})$ for any  $n\in \mathbb{N}$. It turns out that a transitive system
$n\in \mathbb{N}$. It turns out that a transitive system 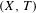 $(X,T)$ is disjoint from all minimal systems if and only if the hyperspace system
$(X,T)$ is disjoint from all minimal systems if and only if the hyperspace system 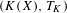 $(K(X),T_{K})$ is disjoint from all minimal systems.
$(K(X),T_{K})$ is disjoint from all minimal systems.
MSC classification
Information
- Type
- Original Article
- Information
- Copyright
- © Cambridge University Press, 2019
References
- 4
- Cited by


