Article contents
Spins of prime ideals and the negative Pell equation  $x^{2}-2py^{2}=-1$
$x^{2}-2py^{2}=-1$
Published online by Cambridge University Press: 23 November 2018
Abstract
Let 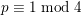 $p\equiv 1\hspace{0.2em}{\rm mod}\hspace{0.2em}4$ be a prime number. We use a number field variant of Vinogradov’s method to prove density results about the following four arithmetic invariants: (i)
$p\equiv 1\hspace{0.2em}{\rm mod}\hspace{0.2em}4$ be a prime number. We use a number field variant of Vinogradov’s method to prove density results about the following four arithmetic invariants: (i)  $16$-rank of the class group
$16$-rank of the class group 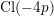 $\text{Cl}(-4p)$ of the imaginary quadratic number field
$\text{Cl}(-4p)$ of the imaginary quadratic number field  $\mathbb{Q}(\sqrt{-4p})$; (ii)
$\mathbb{Q}(\sqrt{-4p})$; (ii) 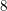 $8$-rank of the ordinary class group
$8$-rank of the ordinary class group 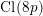 $\text{Cl}(8p)$ of the real quadratic field
$\text{Cl}(8p)$ of the real quadratic field 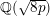 $\mathbb{Q}(\sqrt{8p})$; (iii) the solvability of the negative Pell equation
$\mathbb{Q}(\sqrt{8p})$; (iii) the solvability of the negative Pell equation 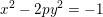 $x^{2}-2py^{2}=-1$ over the integers; (iv)
$x^{2}-2py^{2}=-1$ over the integers; (iv)  $2$-part of the Tate–Šafarevič group
$2$-part of the Tate–Šafarevič group  $\unicode[STIX]{x0428}(E_{p})$ of the congruent number elliptic curve
$\unicode[STIX]{x0428}(E_{p})$ of the congruent number elliptic curve  $E_{p}:y^{2}=x^{3}-p^{2}x$. Our results are conditional on a standard conjecture about short character sums.
$E_{p}:y^{2}=x^{3}-p^{2}x$. Our results are conditional on a standard conjecture about short character sums.
Information
- Type
- Research Article
- Information
- Copyright
- © The Authors 2018
Footnotes
The second author is supported by ERC grant agreement No. 670239.
References
- 4
- Cited by

