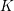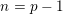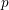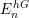Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Mathew, Akhil
2018.
Geometric and Topological Aspects of the Representation Theory of Finite Groups.
Vol. 242,
Issue. ,
p.
269.
Barthel, Tobias
Beaudry, Agnès
and
Stojanoska, Vesna
2019.
Gross–Hopkins duals of higher real K–theory spectra.
Transactions of the American Mathematical Society,
Vol. 372,
Issue. 5,
p.
3347.
Beaudry, Agnès
Bobkova, Irina
Hill, Michael
and
Stojanoska, Vesna
2020.
Invertible K(2)–local E–modules in
C4–spectra.
Algebraic & Geometric Topology,
Vol. 20,
Issue. 7,
p.
3423.
Heard, Drew
Li, Guchuan
and
Shi, XiaoLin Danny
2021.
Picard groups and duality for real Morava
E–theories.
Algebraic & Geometric Topology,
Vol. 21,
Issue. 6,
p.
2703.
Beaudry, Agnès
Goerss, Paul G.
Hopkins, Michael J.
and
Stojanoska, Vesna
2022.
Dualizing spheres for compact p-adic analytic groups and duality in chromatic homotopy.
Inventiones mathematicae,
Vol. 229,
Issue. 3,
p.
1301.
Hahn, Jeremy
Senger, Andrew
and
Wilson, Dylan
2023.
Odd primary analogs of real orientations.
Geometry & Topology,
Vol. 27,
Issue. 1,
p.
87.
Carmeli, Shachar
2023.
On the strict Picard spectrum of commutative ring spectra.
Compositio Mathematica,
Vol. 159,
Issue. 9,
p.
1872.
Mor, Itamar
2024.
The Relative Brauer Group of K(1)-Local Spectra.
International Mathematics Research Notices,
Vol. 2024,
Issue. 15,
p.
11292.
Land, Markus
Mathew, Akhil
Meier, Lennart
and
Tamme, Georg
2024.
Purity in chromatically localized algebraic 𝐾-theory.
Journal of the American Mathematical Society,
Antieau, Benjamin
Meier, Lennart
and
Stojanoska, Vesna
2024.
Picard sheaves, local Brauer groups, and topological modular forms.
Journal of Topology,
Vol. 17,
Issue. 2,
Barthel, Tobias
Castellana, Natàlia
Heard, Drew
Naumann, Niko
Pol, Luca
and
Sanders, Beren
2024.
Triangulated Categories in Representation Theory and Beyond.
Vol. 17,
Issue. ,
p.
1.
Bobkova, Irina
Lachmann, Andrea
Li, Ang
Lima, Alicia
Stojanoska, Vesna
and
Zhang, Adela YiYu
2025.
Bounding the K(p − 1)-local exotic Picard group at p > 3.
Topology and its Applications,
p.
109445.