Published online by Cambridge University Press: 24 June 2016
For a prime number 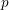 $p$ , we show that differentials
$p$ , we show that differentials 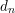 $d_{n}$ in the motivic cohomology spectral sequence with
$d_{n}$ in the motivic cohomology spectral sequence with 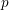 $p$ -local coefficients vanish unless
$p$ -local coefficients vanish unless 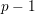 $p-1$ divides
$p-1$ divides  $n-1$ . We obtain an explicit formula for the first non-trivial differential
$n-1$ . We obtain an explicit formula for the first non-trivial differential 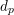 $d_{p}$ , expressing it in terms of motivic Steenrod
$d_{p}$ , expressing it in terms of motivic Steenrod  $p$ -power operations and Bockstein maps. To this end, we compute the algebra of operations of weight
$p$ -power operations and Bockstein maps. To this end, we compute the algebra of operations of weight 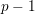 $p-1$ with
$p-1$ with 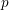 $p$ -local coefficients. Finally, we construct examples of varieties having non-trivial differentials
$p$ -local coefficients. Finally, we construct examples of varieties having non-trivial differentials 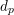 $d_{p}$ in their motivic cohomology spectral sequences.
$d_{p}$ in their motivic cohomology spectral sequences.