Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Déserti, Julie
2015.
On solvable subgroups of the Cremona group.
Illinois Journal of Mathematics,
Vol. 59,
Issue. 2,
Déserti, Julie
2015.
Some properties of the group of birational maps generated by the automorphisms of $${\mathbb {P}}^n_{\mathbb {C}}$$ P C n and the standard involution.
Mathematische Zeitschrift,
Vol. 281,
Issue. 3-4,
p.
893.
Blanc, Jérémy
and
Calabri, Alberto
2016.
On degenerations of plane Cremona transformations.
Mathematische Zeitschrift,
Vol. 282,
Issue. 1-2,
p.
223.
Popov, Vladimir Leonidovich
2019.
Три сюжета о группах Кремоны.
Известия Российской академии наук. Серия математическая,
Vol. 83,
Issue. 4,
p.
194.
Popov, V. L.
2019.
Three plots about the Cremona groups.
Izvestiya: Mathematics,
Vol. 83,
Issue. 4,
p.
830.
Javanpeykar, Ariyan
and
Xie, Junyi
2022.
Finiteness Properties of Pseudo-Hyperbolic Varieties.
International Mathematics Research Notices,
Vol. 2022,
Issue. 3,
p.
1601.
Karzhemanov, Ilya
2024.
On unirational quartic hypersurfaces.
Research in the Mathematical Sciences,
Vol. 11,
Issue. 2,
Bell, Jason
Moosa, Rahim
and
Satriano, Matthew
2024.
On invariant rational functions under rational transformations.
Selecta Mathematica,
Vol. 30,
Issue. 3,
Xie, Junyi
and
Yang, She
2025.
On the Dynamical Mordell–Lang Conjecture in Positive Characteristic.
International Mathematics Research Notices,
Vol. 2025,
Issue. 9,
Regeta, Andriy
Urech, Christian
and
van Santen, Immanuel
2025.
Group theoretical characterizations of rationality.
Inventiones mathematicae,
Yang, She
2025.
A regularization theorem for bounded-degree self-maps.
Science China Mathematics,
Vol. 68,
Issue. 7,
p.
1607.
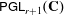 $\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}{\sf PGL}_{r+1}(\mathbf{C})$ to the group
$\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}{\sf PGL}_{r+1}(\mathbf{C})$ to the group 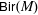 ${\sf Bir}(M)$ of birational transformations of a complex projective variety
${\sf Bir}(M)$ of birational transformations of a complex projective variety 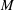 $M$, provided that
$M$, provided that 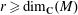 $r\geq \dim _\mathbf{C}(M)$. As a byproduct, we show that: (i)
$r\geq \dim _\mathbf{C}(M)$. As a byproduct, we show that: (i) 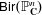 ${\sf Bir}(\mathbb{P}^n_\mathbf{C})$ is isomorphic, as an abstract group, to
${\sf Bir}(\mathbb{P}^n_\mathbf{C})$ is isomorphic, as an abstract group, to 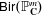 ${\sf Bir}(\mathbb{P}^m_\mathbf{C})$ if and only if
${\sf Bir}(\mathbb{P}^m_\mathbf{C})$ if and only if 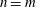 $n=m$; and (ii)
$n=m$; and (ii) 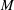 $M$ is rational if and only if
$M$ is rational if and only if 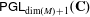 ${\sf PGL}_{\dim (M)+1}(\mathbf{C})$ embeds as a subgroup of
${\sf PGL}_{\dim (M)+1}(\mathbf{C})$ embeds as a subgroup of 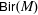 ${\sf Bir}(M)$.
${\sf Bir}(M)$.