Published online by Cambridge University Press: 19 June 2013
The problem of resolution of singularities in positive characteristic can be reformulated as follows: fix a hypersurface 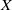 $X$, embedded in a smooth scheme, with points of multiplicity at most
$X$, embedded in a smooth scheme, with points of multiplicity at most  $n$. Let an
$n$. Let an  $n$-sequence of transformations of
$n$-sequence of transformations of 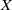 $X$ be a finite composition of monoidal transformations with centers included in the
$X$ be a finite composition of monoidal transformations with centers included in the  $n$-fold points of
$n$-fold points of 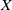 $X$, and of its successive strict transforms. The open problem (in positive characteristic) is to prove that there is an
$X$, and of its successive strict transforms. The open problem (in positive characteristic) is to prove that there is an  $n$-sequence such that the final strict transform of
$n$-sequence such that the final strict transform of 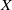 $X$ has no points of multiplicity
$X$ has no points of multiplicity  $n$ (no
$n$ (no  $n$-fold points). In characteristic zero, such an
$n$-fold points). In characteristic zero, such an  $n$-sequence is defined in two steps. The first consists of the transformation of
$n$-sequence is defined in two steps. The first consists of the transformation of  $X$ to a hypersurface with
$X$ to a hypersurface with  $n$-fold points in the so-called monomial case. The second step consists of the elimination of these
$n$-fold points in the so-called monomial case. The second step consists of the elimination of these  $n$-fold points (in the monomial case), which is achieved by a simple combinatorial procedure for choices of centers. The invariants treated in this work allow us to present a notion of strong monomial case which parallels that of monomial case in characteristic zero: if a hypersurface is within the strong monomial case we prove that a resolution can be achieved in a combinatorial manner.
$n$-fold points (in the monomial case), which is achieved by a simple combinatorial procedure for choices of centers. The invariants treated in this work allow us to present a notion of strong monomial case which parallels that of monomial case in characteristic zero: if a hypersurface is within the strong monomial case we prove that a resolution can be achieved in a combinatorial manner.