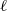 $\ell $-adic Galois representations over function fields
$\ell $-adic Galois representations over function fieldsPublished online by Cambridge University Press: 25 April 2013
Let 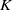 $K$ be a finitely generated extension of
$K$ be a finitely generated extension of  $\mathbb {Q}$. We consider the family of
$\mathbb {Q}$. We consider the family of 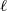 $\ell $-adic representations (
$\ell $-adic representations (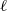 $\ell $ varies through the set of all prime numbers) of the absolute Galois group of
$\ell $ varies through the set of all prime numbers) of the absolute Galois group of 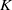 $K$, attached to
$K$, attached to 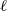 $\ell $-adic cohomology of a separated scheme of finite type over
$\ell $-adic cohomology of a separated scheme of finite type over  $K$. We prove that the fields cut out from the algebraic closure of
$K$. We prove that the fields cut out from the algebraic closure of 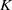 $K$by the kernels of the representations of the family are linearly disjoint over a finite extension of K. This gives a positive answer to a question of Serre.
$K$by the kernels of the representations of the family are linearly disjoint over a finite extension of K. This gives a positive answer to a question of Serre.