Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Manion, Andrew
Marengon, Marco
and
Willis, Michael
2020.
Strands algebras and Ozsváth and Szabó’s
Kauffman-states functor.
Algebraic & Geometric Topology,
Vol. 20,
Issue. 7,
p.
3607.
Azam, Haniya
Cannizzo, Catherine
and
Lee, Heather
2021.
Research Directions in Symplectic and Contact Geometry and Topology.
Vol. 27,
Issue. ,
p.
159.
Dyckerhoff, Tobias
Jasso, Gustavo
and
Lekili, Yankι
2021.
The symplectic geometry of higher Auslander algebras: Symmetric products of disks.
Forum of Mathematics, Sigma,
Vol. 9,
Issue. ,
Lekili, Yankı
and
Ueda, Kazushi
2022.
Homological mirror symmetry for Milnor fibers via moduli of A∞$A_\infty$‐structures.
Journal of Topology,
Vol. 15,
Issue. 3,
p.
1058.
Bai, Shaoyun
and
Côté, Laurent
2023.
On the Rouquier dimension of wrapped Fukaya categories and a conjecture of Orlov.
Compositio Mathematica,
Vol. 159,
Issue. 3,
p.
437.
Chang, William
and
Manion, Andrew
2023.
Compatibility in Ozsváth–Szabó’s bordered
HFK via higher representations.
Pacific Journal of Mathematics,
Vol. 323,
Issue. 2,
p.
253.
Kotelskiy, Artem
Watson, Liam
and
Zibrowius, Claudius
2023.
A mnemonic for the Lipshitz–Ozsváth–Thurston
correspondence.
Algebraic & Geometric Topology,
Vol. 23,
Issue. 6,
p.
2519.
Lekili, Yankı
and
Polishchuk, Alexander
2023.
Homological mirror symmetry for the symmetric squares of punctured spheres.
Advances in Mathematics,
Vol. 418,
Issue. ,
p.
108942.
Hanlon, Andrew
and
Hicks, Jeff
2024.
A short computation of the Rouquier dimension for a cycle of projective lines.
Proceedings of the American Mathematical Society, Series B,
Vol. 11,
Issue. 56,
p.
653.
Gammage, Benjamin
2024.
Mirror symmetry for Berglund-Hübsch Milnor fibers.
Advances in Mathematics,
Vol. 443,
Issue. ,
p.
109563.
Abouzaid, Mohammed
and
Auroux, Denis
2024.
Homological mirror symmetry for hypersurfaces
in (ℂ∗)n.
Geometry & Topology,
Vol. 28,
Issue. 6,
p.
2825.
Lekili, Yankı
and
Ueda, Kazushi
2024.
On homological mirror symmetry for the complement of a smooth ample divisor in a K3 surface.
Kyoto Journal of Mathematics,
Vol. 64,
Issue. 2,
Lauda, Aaron D.
Licata, Anthony M.
and
Manion, Andrew
2024.
From hypertoric geometry to bordered Floer homology via the $$m=1$$ amplituhedron.
Selecta Mathematica,
Vol. 30,
Issue. 3,
Gammage, Benjamin
2024.
Local mirror symmetry via SYZ.
Bulletin of the London Mathematical Society,
Vol. 56,
Issue. 10,
p.
3181.
Lekili, Y.
and
Segal, E.
2025.
Equivariant Fukaya categories at singular values.
Glasgow Mathematical Journal,
p.
1.
 $k+1$ generic hyperplanes in
$k+1$ generic hyperplanes in 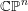 $\mathbb{CP}^{n}$, for
$\mathbb{CP}^{n}$, for 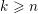 $k\geqslant n$, with respect to certain stops in terms of the endomorphism algebra of a generating set of objects. The stops are chosen so that the resulting algebra is formal. In the case of the complement of
$k\geqslant n$, with respect to certain stops in terms of the endomorphism algebra of a generating set of objects. The stops are chosen so that the resulting algebra is formal. In the case of the complement of  $n+2$ generic hyperplanes in
$n+2$ generic hyperplanes in 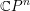 $\mathbb{C}P^{n}$ (
$\mathbb{C}P^{n}$ ( $n$-dimensional pair of pants), we show that our partial wrapped Fukaya category is equivalent to a certain categorical resolution of the derived category of the singular affine variety
$n$-dimensional pair of pants), we show that our partial wrapped Fukaya category is equivalent to a certain categorical resolution of the derived category of the singular affine variety 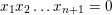 $x_{1}x_{2}\ldots x_{n+1}=0$. By localizing, we deduce that the (fully) wrapped Fukaya category of the
$x_{1}x_{2}\ldots x_{n+1}=0$. By localizing, we deduce that the (fully) wrapped Fukaya category of the  $n$-dimensional pair of pants is equivalent to the derived category of
$n$-dimensional pair of pants is equivalent to the derived category of 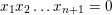 $x_{1}x_{2}\ldots x_{n+1}=0$. We also prove similar equivalences for finite abelian covers of the
$x_{1}x_{2}\ldots x_{n+1}=0$. We also prove similar equivalences for finite abelian covers of the  $n$-dimensional pair of pants.
$n$-dimensional pair of pants.
