Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Brugallé, Erwan
and
Shaw, Kristin
2015.
Obstructions to Approximating Tropical Curves in Surfaces Via Intersection Theory.
Canadian Journal of Mathematics,
Vol. 67,
Issue. 3,
p.
527.
Ongaro, Jared
and
Shapiro, Boris
2015.
A Note on Planarity Stratification of Hurwitz Spaces.
Canadian Mathematical Bulletin,
Vol. 58,
Issue. 3,
p.
596.
Brugallé, Erwan
and
Markwig, Hannah
2016.
Deformation of tropical Hirzebruch surfaces and enumerative geometry.
Journal of Algebraic Geometry,
Vol. 25,
Issue. 4,
p.
633.
Bertram, Aaron
and
Easton, Robert
2017.
The tropical Nullstellensatz for congruences.
Advances in Mathematics,
Vol. 308,
Issue. ,
p.
36.
Ardila, Federico
and
Brugallé, Erwan
2017.
The Double Gromov–Witten Invariants of Hirzebruch Surfaces are Piecewise Polynomial.
International Mathematics Research Notices,
Vol. 2017,
Issue. 2,
p.
614.
Gathmann, Andreas
Markwig, Hannah
and
Ochse, Dennis
2017.
Algorithmic and Experimental Methods in Algebra, Geometry, and Number Theory.
p.
287.
Cools, Filip
and
Draisma, Jan
2018.
On metric graphs with prescribed gonality.
Journal of Combinatorial Theory, Series A,
Vol. 156,
Issue. ,
p.
1.
Markwig, Hannah
Markwig, Thomas
and
Shustin, Eugenii
2018.
Enumeration of complex and real surfaces via tropical geometry.
Advances in Geometry ,
Vol. 18,
Issue. 1,
p.
69.
Ilten, Nathan
and
Len, Yoav
2019.
Projective duals to algebraic and tropical hypersurfaces.
Proceedings of the London Mathematical Society,
Vol. 119,
Issue. 5,
p.
1234.
Ranganathan, Dhruv
Santos-Parker, Keli
and
Wise, Jonathan
2019.
Moduli of stable maps in genus one and logarithmic geometry, II.
Algebra & Number Theory,
Vol. 13,
Issue. 8,
p.
1765.
Markwig, Hannah
2020.
Tropical Curves and Covers and Their Moduli Spaces.
Jahresbericht der Deutschen Mathematiker-Vereinigung,
Vol. 122,
Issue. 3,
p.
139.
Kazarnovskii, B. Ya.
Khovanskii, A. G.
and
Esterov, A. I.
2021.
Newton polytopes and tropical geometry.
Russian Mathematical Surveys,
Vol. 76,
Issue. 1,
p.
91.
Kazarnovskii, Boris Yakovlevich
Khovanskii, Askold Georgievich
and
Esterov, Alexander Isaakovich
2021.
Многогранники Ньютона и тропическая геометрия.
Успехи математических наук,
Vol. 76,
Issue. 1(457),
p.
95.
Blomme, Thomas
and
Markwig, Hannah
2023.
Tropical descendant invariants with line constraints.
Journal of the London Mathematical Society,
Vol. 108,
Issue. 6,
p.
2248.
Brugallé, Erwan
2024.
Géométries énumératives complexe, réelle et tropicale.
Journées mathématiques X-UPS,
p.
27.
Corey, Daniel
Markwig, Hannah
and
Ranganathan, Dhruv
2025.
Counting Tropical Curves in ℙ1 × ℙ1 : Computation and Polynomiality Properties.
International Mathematics Research Notices,
Vol. 2025,
Issue. 15,
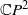 $ \mathbb{C} {P}^{2} $ is a classical problem of enumerative geometry posed by Zeuthen more than a century ago. For a given
$ \mathbb{C} {P}^{2} $ is a classical problem of enumerative geometry posed by Zeuthen more than a century ago. For a given 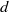 $d$ and
$d$ and 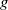 $g$ one has to find the number of degree
$g$ one has to find the number of degree 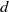 $d$ genus
$d$ genus 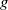 $g$ curves that pass through a certain generic configuration of points and at the same time are tangent to a certain generic configuration of lines. The total number of points and lines in these two configurations is
$g$ curves that pass through a certain generic configuration of points and at the same time are tangent to a certain generic configuration of lines. The total number of points and lines in these two configurations is 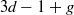 $3d- 1+ g$ so that the answer is a finite integer number. In this paper we translate this classical problem to the corresponding enumerative problem of tropical geometry in the case when
$3d- 1+ g$ so that the answer is a finite integer number. In this paper we translate this classical problem to the corresponding enumerative problem of tropical geometry in the case when 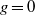 $g= 0$. Namely, we show that the tropical problem is well posed and establish a special case of the correspondence theorem that ensures that the corresponding tropical and classical numbers coincide. Then we use the floor diagram calculus to reduce the problem to pure combinatorics. As a consequence, we express genus 0 characteristic numbers of
$g= 0$. Namely, we show that the tropical problem is well posed and establish a special case of the correspondence theorem that ensures that the corresponding tropical and classical numbers coincide. Then we use the floor diagram calculus to reduce the problem to pure combinatorics. As a consequence, we express genus 0 characteristic numbers of 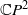 $ \mathbb{C} {P}^{2} $ in terms of open Hurwitz numbers.
$ \mathbb{C} {P}^{2} $ in terms of open Hurwitz numbers.