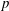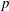Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Wood, Melanie Machett
and
Yasuda, Takehiko
2015.
Mass Formulas for Local Galois Representations and Quotient Singularities. I: A Comparison of Counting Functions.
International Mathematics Research Notices,
p.
rnv074.
YASUDA, TAKEHIKO
2016.
WILDER MCKAY CORRESPONDENCES.
Nagoya Mathematical Journal,
Vol. 221,
Issue. 1,
p.
111.
Wood, Melanie
and
Yasuda, Takehiko
2017.
Mass formulas for local Galois representations and quotient singularities II: Dualities and resolution of singularities.
Algebra & Number Theory,
Vol. 11,
Issue. 4,
p.
817.
Totaro, Burt
2019.
The failure of Kodaira vanishing for Fano varieties, and terminal singularities that are not Cohen-Macaulay.
Journal of Algebraic Geometry,
Vol. 28,
Issue. 4,
p.
751.
Tonini, Fabio
and
Yasuda, Takehiko
2020.
Moduli of formal torsors.
Journal of Algebraic Geometry,
Vol. 29,
Issue. 4,
p.
753.
León-Cardenal, Edwin
Martín-Morales, Jorge
Veys, Willem
and
Viu-Sos, Juan
2020.
Motivic zeta functions on Q-Gorenstein varieties.
Advances in Mathematics,
Vol. 370,
Issue. ,
p.
107192.
Chen, Yin
Du, Rong
and
Gao, Yun
2020.
Modular quotient varieties and singularities by the cyclic group of order 2p.
Communications in Algebra,
Vol. 48,
Issue. 12,
p.
5490.
Yamamoto, Takahiro
2021.
Pathological Quotient Singularities in Characteristic Three Which Are Not Log Canonical.
Michigan Mathematical Journal,
Vol. 70,
Issue. 4,
Tanno, Mahito
and
Yasuda, Takehiko
2021.
The wild McKay correspondence for cyclic groups of prime power order.
Illinois Journal of Mathematics,
Vol. 65,
Issue. 3,
Yasuda, Takehiko
and
the author
2022.
The wild McKay correspondence via motivic integration.
Sugaku Expositions,
Vol. 35,
Issue. 1,
p.
1.
Tanno, Mahito
2022.
On convergence of stringy motives of wild pn-cyclic quotient singularities.
Communications in Algebra,
Vol. 50,
Issue. 5,
p.
2184.
Fan, Linghu
2023.
Crepant resolution of $\mathbf{A}^{4}/A_{4}$ in characteristic 2.
Proceedings of the Japan Academy, Series A, Mathematical Sciences,
Vol. 99,
Issue. 9,
Bernasconi, Fabio
and
Kollár, János
2023.
Vanishing Theorems for Three-folds in Characteristicp> 5.
International Mathematics Research Notices,
Vol. 2023,
Issue. 4,
p.
2846.