Published online by Cambridge University Press: 10 May 2013
Let 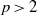 $p\gt 2$ be prime, and let
$p\gt 2$ be prime, and let  $F$ be a totally real field in which
$F$ be a totally real field in which 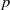 $p$ is unramified. We give a sufficient criterion for a
$p$ is unramified. We give a sufficient criterion for a 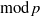 $\mathrm{mod} \hspace{0.167em} p$ Galois representation to arise from a
$\mathrm{mod} \hspace{0.167em} p$ Galois representation to arise from a 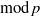 $\mathrm{mod} \hspace{0.167em} p$ Hilbert modular form of parallel weight one, by proving a ‘companion forms’ theorem in this case. The techniques used are a mixture of modularity lifting theorems and geometric methods. As an application, we show that Serre’s conjecture for
$\mathrm{mod} \hspace{0.167em} p$ Hilbert modular form of parallel weight one, by proving a ‘companion forms’ theorem in this case. The techniques used are a mixture of modularity lifting theorems and geometric methods. As an application, we show that Serre’s conjecture for 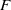 $F$ implies Artin’s conjecture for totally odd two-dimensional representations over
$F$ implies Artin’s conjecture for totally odd two-dimensional representations over 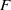 $F$.
$F$.