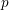Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
LIU, JI-CAI
and
WANG, CHEN
2019.
CONGRUENCES FOR THE TH APÉRY NUMBER.
Bulletin of the Australian Mathematical Society,
Vol. 99,
Issue. 03,
p.
362.
Gomez, Alexis
McCarthy, Dermot
and
Young, Dylan
2019.
Apéry-like numbers and families of newforms with complex multiplication.
Research in Number Theory,
Vol. 5,
Issue. 1,
Henningsen, Joel A.
and
Straub, Armin
2022.
Generalized Lucas congruences and linear p-schemes.
Advances in Applied Mathematics,
Vol. 141,
Issue. ,
p.
102409.
Gorodetsky, Ofir
2023.
New Representations for all Sporadic Apéry-Like Sequences, With Applications to Congruences.
Experimental Mathematics,
Vol. 32,
Issue. 4,
p.
641.
Straub, Armin
2024.
Gessel–Lucas congruences for sporadic sequences.
Monatshefte für Mathematik,
Vol. 203,
Issue. 4,
p.
883.
Beukers, Frits
Tsai, Wei‐Lun
and
Ye, Dongxi
2025.
Lucas congruences using modular forms.
Bulletin of the London Mathematical Society,
Vol. 57,
Issue. 1,
p.
69.