Published online by Cambridge University Press: 08 February 2022
We prove the generic Torelli theorem for hypersurfaces in 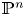 $\mathbb {P}^{n}$ of degree
$\mathbb {P}^{n}$ of degree  $d$ dividing
$d$ dividing 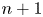 $n+1$, for
$n+1$, for  $d$ sufficiently large. Our proof involves the higher-order study of the variation of Hodge structure along particular one-parameter families of hypersurfaces that we call ‘Schiffer variations.’ We also analyze the case of degree
$d$ sufficiently large. Our proof involves the higher-order study of the variation of Hodge structure along particular one-parameter families of hypersurfaces that we call ‘Schiffer variations.’ We also analyze the case of degree  $4$. Combined with Donagi's generic Torelli theorem and results of Cox and Green, this shows that the generic Torelli theorem for hypersurfaces holds with finitely many exceptions.
$4$. Combined with Donagi's generic Torelli theorem and results of Cox and Green, this shows that the generic Torelli theorem for hypersurfaces holds with finitely many exceptions.
The author is supported by the ERC Synergy Grant HyperK (Grant agreement No. 854361).