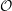 ${\mathcal{O}}$ for quantized symplectic resolutions
${\mathcal{O}}$ for quantized symplectic resolutionsPublished online by Cambridge University Press: 07 September 2017
In this paper we study categories 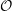 ${\mathcal{O}}$ over quantizations of symplectic resolutions admitting Hamiltonian tori actions with finitely many fixed points. In this generality, these categories were introduced by Braden et al. We establish a family of standardly stratified structures (in the sense of the author and Webster) on these categories
${\mathcal{O}}$ over quantizations of symplectic resolutions admitting Hamiltonian tori actions with finitely many fixed points. In this generality, these categories were introduced by Braden et al. We establish a family of standardly stratified structures (in the sense of the author and Webster) on these categories 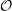 ${\mathcal{O}}$ . We use these structures to study shuffling functors of Braden et al. (called cross-walling functors in this paper). Most importantly, we prove that all cross-walling functors are derived equivalences that define an action of the Deligne groupoid of a suitable real hyperplane arrangement.
${\mathcal{O}}$ . We use these structures to study shuffling functors of Braden et al. (called cross-walling functors in this paper). Most importantly, we prove that all cross-walling functors are derived equivalences that define an action of the Deligne groupoid of a suitable real hyperplane arrangement.
To send this article to your Kindle, first ensure no-reply@cambridge.org is added to your Approved Personal Document E-mail List under your Personal Document Settings on the Manage Your Content and Devices page of your Amazon account. Then enter the ‘name’ part of your Kindle email address below. Find out more about sending to your Kindle. Find out more about saving to your Kindle.
Note you can select to save to either the @free.kindle.com or @kindle.com variations. ‘@free.kindle.com’ emails are free but can only be saved to your device when it is connected to wi-fi. ‘@kindle.com’ emails can be delivered even when you are not connected to wi-fi, but note that service fees apply.
Find out more about the Kindle Personal Document Service.
 ${\mathcal{O}}$ for quantized symplectic resolutions
${\mathcal{O}}$ for quantized symplectic resolutionsTo save this article to your Dropbox account, please select one or more formats and confirm that you agree to abide by our usage policies. If this is the first time you used this feature, you will be asked to authorise Cambridge Core to connect with your Dropbox account. Find out more about saving content to Dropbox.
 ${\mathcal{O}}$ for quantized symplectic resolutions
${\mathcal{O}}$ for quantized symplectic resolutionsTo save this article to your Google Drive account, please select one or more formats and confirm that you agree to abide by our usage policies. If this is the first time you used this feature, you will be asked to authorise Cambridge Core to connect with your Google Drive account. Find out more about saving content to Google Drive.
 ${\mathcal{O}}$ for quantized symplectic resolutions
${\mathcal{O}}$ for quantized symplectic resolutions