Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Bellaïche, Joël
2015.
Unitary Eigenvarieties at Isobaric Points.
Canadian Journal of Mathematics,
Vol. 67,
Issue. 2,
p.
315.
Patrikis, Stefan
2016.
Generalized Kuga–Satake theory and rigid local systems, II : rigid Hecke eigensheaves.
Algebra & Number Theory,
Vol. 10,
Issue. 7,
p.
1477.
Xia, Yuhou
2019.
Irreducibility of automorphic Galois representations of low dimensions.
Mathematische Annalen,
Vol. 374,
Issue. 3-4,
p.
1953.
Goresky, Mark
and
Tai, Yung sheng
2019.
Ordinary points mod p of GLn(ℝ)-locally
symmetric spaces.
Pacific Journal of Mathematics,
Vol. 303,
Issue. 1,
p.
165.
Guiraud, David-Alexandre
2020.
Unobstructedness of Galois deformation rings associated to regular algebraic conjugate self-dual cuspidal automorphic representations.
Algebra & Number Theory,
Vol. 14,
Issue. 6,
p.
1331.
Amadio Guidi, Federico
2021.
Independence of algebraic monodromy groups in compatible systems.
Selecta Mathematica,
Vol. 27,
Issue. 4,
Boxer, George
Calegari, Frank
Gee, Toby
and
Pilloni, Vincent
2021.
Abelian surfaces over totally real fields are potentially modular.
Publications mathématiques de l'IHÉS,
Vol. 134,
Issue. 1,
p.
153.
Calegari, Frank
Emerton, Matthew
and
Gee, Toby
2022.
GLOBALLY REALIZABLE COMPONENTS OF LOCAL DEFORMATION RINGS.
Journal of the Institute of Mathematics of Jussieu,
Vol. 21,
Issue. 2,
p.
533.
Weiss, Ariel
2022.
On the images of Galois representations attached to low weight Siegel modular forms.
Journal of the London Mathematical Society,
Vol. 106,
Issue. 1,
p.
358.
Fresán, Javier
Sabbah, Claude
and
Yu, Jeng-Daw
2022.
Hodge theory of Kloosterman connections.
Duke Mathematical Journal,
Vol. 171,
Issue. 8,
Sabbah, Claude
and
Yu, Jeng-Daw
2023.
Hodge properties of Airy moments.
Tunisian Journal of Mathematics,
Vol. 5,
Issue. 2,
p.
215.
Hui, Chun Yin
2023.
Monodromy of four‐dimensional irreducible compatible systems of Q$\mathbb {Q}$.
Bulletin of the London Mathematical Society,
Vol. 55,
Issue. 4,
p.
1773.
Golyshev, Vasily
and
Clingher, Adrian
2023.
On L-derivatives and biextensions of Calabi–Yau motives.
Experimental Results,
Vol. 4,
Issue. ,
Hui, Chun Yin
2023.
Monodromy of subrepresentations and irreducibility of low degree automorphic Galois representations.
Journal of the London Mathematical Society,
Vol. 108,
Issue. 6,
p.
2436.
Kret, Arno
and
Shin, Sug Woo
2024.
GALOIS REPRESENTATIONS FOR EVEN GENERAL SPECIAL ORTHOGONAL GROUPS.
Journal of the Institute of Mathematics of Jussieu,
Vol. 23,
Issue. 5,
p.
1959.
Qin, Yichen
2024.
L${L}$‐functions of Kloosterman sheaves.
Proceedings of the London Mathematical Society,
Vol. 129,
Issue. 5,
Wu, Ju-Feng
2024.
On adjoint Bloch–Kato Selmer groups for $$\textrm{GSp}_{2g}$$.
Annales mathématiques du Québec,
Vol. 48,
Issue. 1,
p.
187.
Qin, Yichen
2024.
Hodge numbers of motives attached to Kloosterman and Airy moments.
Journal für die reine und angewandte Mathematik (Crelles Journal),
Vol. 0,
Issue. 0,
Mochizuki, Takuro
2025.
Perspectives on Four Decades of Algebraic Geometry, Volume 2.
Vol. 352,
Issue. ,
p.
13.
 $l$-adic representations is potentially automorphic. The innovation is that we make no irreducibility assumption, but we make a purity assumption instead. For compatible systems coming from geometry, purity is often easier to check than irreducibility. We use Katz’s theory of rigid local systems to construct many examples of motives to which our theorem applies. We also show that if
$l$-adic representations is potentially automorphic. The innovation is that we make no irreducibility assumption, but we make a purity assumption instead. For compatible systems coming from geometry, purity is often easier to check than irreducibility. We use Katz’s theory of rigid local systems to construct many examples of motives to which our theorem applies. We also show that if  $F$ is a CM or totally real field and if
$F$ is a CM or totally real field and if  ${\it\pi}$ is a polarizable, regular algebraic, cuspidal automorphic representation of
${\it\pi}$ is a polarizable, regular algebraic, cuspidal automorphic representation of 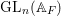 $\text{GL}_{n}(\mathbb{A}_{F})$, then for a positive Dirichlet density set of rational primes
$\text{GL}_{n}(\mathbb{A}_{F})$, then for a positive Dirichlet density set of rational primes 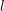 $l$, the
$l$, the  $l$-adic representations
$l$-adic representations 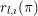 $r_{l,\imath }({\it\pi})$ associated to
$r_{l,\imath }({\it\pi})$ associated to  ${\it\pi}$ are irreducible.
${\it\pi}$ are irreducible.
