Article contents
Affine cluster monomials are generalized minors
Published online by Cambridge University Press: 14 June 2019
Abstract
We study the realization of acyclic cluster algebras as coordinate rings of Coxeter double Bruhat cells in Kac–Moody groups. We prove that all cluster monomials with 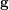 $\mathbf{g}$-vector lying in the doubled Cambrian fan are restrictions of principal generalized minors. As a corollary, cluster algebras of finite and affine type admit a complete and non-recursive description via (ind-)algebraic group representations, in a way similar in spirit to the Caldero–Chapoton description via quiver representations. In type
$\mathbf{g}$-vector lying in the doubled Cambrian fan are restrictions of principal generalized minors. As a corollary, cluster algebras of finite and affine type admit a complete and non-recursive description via (ind-)algebraic group representations, in a way similar in spirit to the Caldero–Chapoton description via quiver representations. In type 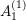 $A_{1}^{(1)}$, we further show that elements of several canonical bases (generic, triangular, and theta) which complete the partial basis of cluster monomials are composed entirely of restrictions of minors. The discrepancy among these bases is accounted for by continuous parameters appearing in the classification of irreducible level-zero representations of affine Lie groups. We discuss how our results illuminate certain parallels between the classification of representations of finite-dimensional algebras and of integrable weight representations of Kac–Moody algebras.
$A_{1}^{(1)}$, we further show that elements of several canonical bases (generic, triangular, and theta) which complete the partial basis of cluster monomials are composed entirely of restrictions of minors. The discrepancy among these bases is accounted for by continuous parameters appearing in the classification of irreducible level-zero representations of affine Lie groups. We discuss how our results illuminate certain parallels between the classification of representations of finite-dimensional algebras and of integrable weight representations of Kac–Moody algebras.
Keywords
MSC classification
Information
- Type
- Research Article
- Information
- Copyright
- © The Authors 2019
References
- 1
- Cited by

