No CrossRef data available.
Article contents
On random walks and switched random walks on homogeneous spaces
Published online by Cambridge University Press: 28 November 2022
Abstract
We prove new mixing rate estimates for the random walks on homogeneous spaces determined by a probability distribution on a finite group 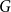 $G$. We introduce the switched random walk determined by a finite set of probability distributions on
$G$. We introduce the switched random walk determined by a finite set of probability distributions on  $G$, prove that its long-term behaviour is determined by the Fourier joint spectral radius of the distributions, and give Hermitian sum-of-squares algorithms for the effective estimation of this quantity.
$G$, prove that its long-term behaviour is determined by the Fourier joint spectral radius of the distributions, and give Hermitian sum-of-squares algorithms for the effective estimation of this quantity.
Keywords
Information
- Type
- Paper
- Information
- Copyright
- © The Author(s), 2022. Published by Cambridge University Press
References
Ahmadi, A. A. and Jungers, R. M. (2016) Lower bounds on complexity of Lyapunov functions for switched linear systems. Nonlinear Anal. Hybrid Syst. 21 118–129. DOI 10.1016/j.nahs.2016.01.003.CrossRefGoogle Scholar
Jungers, R. M., Ahmadi, A. A., Parrilo, P. A. and Roozbehani, M. (2017) A characterization of Lyapunov inequalities for stability of switched systems. IEEE Trans. Automat. Control 62(6) 3062–3067. DOI 10.1109/TAC.2017.2671345.Google Scholar
Diaconis, P. (1988) Group Representations in Probability and Statistics. Institute of Mathematical Statistics, Hayward, CA, Institute of Mathematical Statistics Lecture Notes—Monograph Series, 11,Google Scholar
Boyd, S., Diaconis, P., Parrilo, P. and Xiao, L. (2009) Fastest mixing Markov chain on graphs with symmetries. SIAM J. Optim. 20(2) 792–819. DOI 10.1137/070689413.CrossRefGoogle Scholar
Boyd, S., Diaconis, P. and Xiao, L. (2004) Fastest mixing Markov chain on a graph. SIAM Rev. 46(4) 667–689. DOI 10.1137/S0036144503423264.CrossRefGoogle Scholar
Blekherman, G., Parrilo, P. A. and Thomas, R. R. (2013) Semidefinite Optimization and Convex Algebraic Geometry. Society for Industrial and Applied Mathematics (SIAM), Mathematical Optimization Society, Philadelphia, PA, MOS-SIAM Series on Optimization, 13,Google Scholar
Blondel, V. D. and Tsitsiklis, J. N. (2000) The boundedness of all products of a pair of matrices is undecidable. Syst. Control Lett. 41(2) 135–140. DOI 10.1016/S0167-6911(00)00049-9.CrossRefGoogle Scholar
Ahmadi, A. A., de Klerk, E. and Hall, G. (2019) Polynomial norms. SIAM J. Optim. 29(1) 399–422. DOI 10.1137/18M1172843.CrossRefGoogle Scholar
Curto, R. E. and Fialkow, L. A. (2002) A duality proof of Tchakaloff’s theorem. J. Math. Anal. Appl. 269(2) 519–532. DOI 10.1016/S0022-247X(02)00034-3.CrossRefGoogle Scholar
Diaconis, P. and Shahshahani, M. (1981) Generating a random permutation with random transpositions. Z Wahrsch. Verw. Gebiete 57(2) 159–179. DOI 10.1007/BF00535487.CrossRefGoogle Scholar
Fulton, W. and Harris, J. (1991) Representation Theory, Graduate Texts in Mathematics. Springer-Verlag, New York, A first course; Readings in Mathematics, 129,Google Scholar
Hoeffding, W. (1963) Probability inequalities for sums of bounded random variables. J. Am. Statist. Assoc. 58 13–30.CrossRefGoogle Scholar
Parrilo, P. A. and Jadbabaie, A. (2008) Approximation of the joint spectral radius using sum of squares. Linear Algebra Appl. 428(10) 2385–2402. DOI 10.1016/j.laa.2007.12.027.CrossRefGoogle Scholar
Breuillard, E. On the joint spectral radius, ArXiv preprint, posted on March 2021, https://arxiv.org/abs/2103.09089
Google Scholar
Madras, N. (2002). Lectures on Monte Carlo Methods. American Mathematical Society, Providence, RI, Fields Institute Monographs, 16,Google Scholar
Bernstein, M. (2018) A random walk on the symmetric group generated by random involutions. Electron. J. Probab. 23 PaperNo. 26, 28. DOI 10.1214/18-EJP140.CrossRefGoogle Scholar
Bernstein, M. and Nestoridi, E. (2019) Cutoff for random to random card shuffle. Ann. Probab. 47(5) 3303–3320. DOI 10.1214/19-AOP1340.CrossRefGoogle Scholar
Goldberg, M. and Zwas, G. (1974) On matrices having equal spectral radius and spectral norm. Linear Algebra Appl. 8(5) 427–434. DOI 10.1016/0024-3795(74)90076-7.CrossRefGoogle Scholar
Rota, G.-C. and Strang, G. (1960) A note on the joint spectral radius. Nederl. Akad. Wetensch. Proc. Ser. A 63 = Indag. Math. 22 379–381, 1960.CrossRefGoogle Scholar
Boyd, S., Diaconis, P. and Xiao, L. (2004) Fastest mixing Markov chain on a graph. SIAM Rev. 46(4) 667–689. DOI 10.1137/S0036144503423264.CrossRefGoogle Scholar
Sagan, B. E. (2001)
. The Symmetric Group: Representations, Combinatorial Algorithms, and Symmetric Functions. Springer-Verlag, New York, Graduate Texts in Mathematics, 203, 2nd,CrossRefGoogle Scholar


