No CrossRef data available.
Article contents
VMO Space Associated with Parabolic Sections and its Application
Published online by Cambridge University Press: 20 November 2018
Abstract
Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.
In this paper we define a space  $VM{{O}_{P}}$ associated with a family
$VM{{O}_{P}}$ associated with a family  $P$ of parabolic sections and show that the dual of
$P$ of parabolic sections and show that the dual of  $VM{{O}_{P}}$ is the Hardy space
$VM{{O}_{P}}$ is the Hardy space 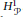 $H_{P}^{1}$ . As an application, we prove that almost everywhere convergence of a bounded sequence in
$H_{P}^{1}$ . As an application, we prove that almost everywhere convergence of a bounded sequence in 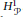 $H_{P}^{1}$ implies weak
$H_{P}^{1}$ implies weak  $^{\star }$ convergence
$^{\star }$ convergence
Information
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 2015
References
[1]
Aimar, H., Forzani, L., and Toledano, R., Balls and quasi-metrics: a space of homogeneous type modeling the real analysis related to the Monge-Ampère equation.
J. Fourier Anal. Appl.
4(1998), no. 4-5, 377–381. http://dx.doi.org/10.1007/BF02498215
Google Scholar
[2]
Caffarelli, L. A., Some regularity properties of solutions of Monge-Ampere equation.
Comm. Pure Appl. Math.
44(1991), no. 8-9, 965–969. http://dx.doi.org/10.1002/cpa.3160440809
Google Scholar
[3]
Caffarelli, L. A. , Boundary regularity of maps with convex potentials.
Comm. Pure Appl. Math.
45(1992), no. 9, 1141–1151. http://dx.doi.org/10.1002/cpa.3160450905
Google Scholar
[4]
Caffarelli, L. A. and Gutierrez, C. E., Real analysis related to the Monge-Ampère equation.
Trans. Amer. Math. Soc.
348(1996), no. 3, 1075–1092. http://dx.doi.org/10.1090/S0002-9947-96-01473-0
Google Scholar
[5]
Caffarelli, L. A. and Gutierrez, C. E., Properties of the solutions of the linearized Monge-Ampère equation.
Amer. J. Math.
119(1997), no. 2, 423–465. http://dx.doi.org/10.1353/ajm.1997.0010
Google Scholar
[6]
Coifman, R. and Weiss, G., Extensions of Hardy spaces and their use in analysis.
Bull. Amer. Math. Soc.
83(1977), no. 4, 569–645. http://dx.doi.org/10.1090/S0002-9904-1977-14325-5
Google Scholar
[7]
Coifman, R., Lions, P.-L., Meyer, Y., and Semmes, S., Compensated compactness and Hardy sapces.
J. Math.Pures Appl.
72(1993), no. 3, 247–286.Google Scholar
[8]
Ding, Y. and Lin, C.-C., Hardy spaces associated to the sections.
Tohoku Math. J.
57(2005), no. 2, 147–170. http://dx.doi.Org/1 0.2748/tmj/111 9888333
Google Scholar
[9]
Huang, Q., Harnack inequality for the linearized parabolic Monge-Ampère equation.
Trans. Amer. Math. Soc.
351(1999), 2025–2054. http://dx.doi.org/10.1090/S0002-9947-99-02142-X
Google Scholar
[10]
Jones, P. W. and Journé,
On weak convergence in H1^).
Proc. Amer. Math. Soc.
120(1994), 137–138.Google Scholar
[11]
Qu, M. and Wu, X., BMO spaces associated to generalized parabolic sections.
Anal.Theory Appl.
27(2011), no. 1, 1–9. http://dx.doi.Org/10.1 007/s1 0496-011-0001 -2
Google Scholar
[12]
Wu, X., Hardy spaces associated to generalized parabolic sections.
Panamer.Math. J.
18(2008), no. 2, 33–51.Google Scholar

