Article contents
The Values of Modular Functions and Modular Forms
Published online by Cambridge University Press: 20 November 2018
Abstract
Let 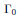 ${{\Gamma }_{0}}$ be a Fuchsian group of the first kind of genus zero and
${{\Gamma }_{0}}$ be a Fuchsian group of the first kind of genus zero and 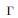 $\Gamma$ be a subgroup of
$\Gamma$ be a subgroup of 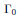 ${{\Gamma }_{0}}$ of finite index of genus zero. We find universal recursive relations giving the
${{\Gamma }_{0}}$ of finite index of genus zero. We find universal recursive relations giving the 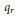 ${{q}_{r}}$ -series coefficients of
${{q}_{r}}$ -series coefficients of  ${{j}_{0}}$ by using those of the
${{j}_{0}}$ by using those of the 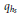 ${{q}_{{{h}_{s}}}}$ -series of
${{q}_{{{h}_{s}}}}$ -series of 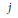 $j$ , where
$j$ , where 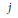 $j$ is the canonical Hauptmodul for
$j$ is the canonical Hauptmodul for 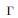 $\Gamma$ and
$\Gamma$ and  ${{j}_{0}}$ is a Hauptmodul for
${{j}_{0}}$ is a Hauptmodul for 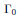 ${{\Gamma }_{0}}$ without zeros on the complex upper half plane
${{\Gamma }_{0}}$ without zeros on the complex upper half plane 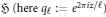 $\mathfrak{H}\left( \text{here}\,\,{{q}_{\ell }}\,:=\,{{e}^{2\pi iz/\ell }} \right)$ . We find universal recursive formulas for
$\mathfrak{H}\left( \text{here}\,\,{{q}_{\ell }}\,:=\,{{e}^{2\pi iz/\ell }} \right)$ . We find universal recursive formulas for 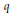 $q$ -series coefficients of any modular form on
$q$ -series coefficients of any modular form on 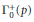 $\Gamma _{0}^{+}\left( p \right)$ in terms of those of the canonical Hauptmodul
$\Gamma _{0}^{+}\left( p \right)$ in terms of those of the canonical Hauptmodul 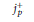 $j_{p}^{+}$ .
$j_{p}^{+}$ .
Information
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 2006
References
- 4
- Cited by

