No CrossRef data available.
Published online by Cambridge University Press: 20 November 2018
We consider homogeneous multiaffine polynomials whose coefficients are the Plücker coordinates of a point 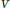 $V$ of the Grassmannian. We show that such a polynomial is stable (with respect to the upper half plane) if and only if
$V$ of the Grassmannian. We show that such a polynomial is stable (with respect to the upper half plane) if and only if 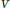 $V$ is in the totally nonnegative part of the Grassmannian. To prove this, we consider an action of matrices on multiaffine polynomials. We show that a matrix
$V$ is in the totally nonnegative part of the Grassmannian. To prove this, we consider an action of matrices on multiaffine polynomials. We show that a matrix  $A$ preserves stability of polynomials if and only if
$A$ preserves stability of polynomials if and only if  $A$ is totally nonnegative. The proofs are applications of classical theory of totally nonnegative matrices, and the generalized Pólya-Schur theory of Borcea and Brändén.
$A$ is totally nonnegative. The proofs are applications of classical theory of totally nonnegative matrices, and the generalized Pólya-Schur theory of Borcea and Brändén.