Published online by Cambridge University Press: 15 November 2018
A Tits polygon is a bipartite graph in which the neighborhood of every vertex is endowed with an “opposition relation” satisfying certain properties. Moufang polygons are precisely the Tits polygons in which these opposition relations are all trivial. There is a standard construction that produces a Tits polygon whose opposition relations are not all trivial from an arbitrary pair 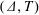 $(\unicode[STIX]{x1D6E5},T)$, where
$(\unicode[STIX]{x1D6E5},T)$, where 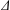 $\unicode[STIX]{x1D6E5}$ is a building of type
$\unicode[STIX]{x1D6E5}$ is a building of type  $\unicode[STIX]{x1D6F1}$,
$\unicode[STIX]{x1D6F1}$, 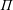 $\unicode[STIX]{x1D6F1}$ is a spherical, irreducible Coxeter diagram of rank at least
$\unicode[STIX]{x1D6F1}$ is a spherical, irreducible Coxeter diagram of rank at least 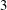 $3$, and
$3$, and 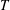 $T$ is a Tits index of absolute type
$T$ is a Tits index of absolute type 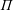 $\unicode[STIX]{x1D6F1}$ and relative rank
$\unicode[STIX]{x1D6F1}$ and relative rank 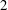 $2$. A Tits polygon is called
$2$. A Tits polygon is called  $k$-plump if its opposition relations satisfy a mild condition that is satisfied by all Tits triangles coming from a pair
$k$-plump if its opposition relations satisfy a mild condition that is satisfied by all Tits triangles coming from a pair  $(\unicode[STIX]{x1D6E5},T)$ such that every panel of
$(\unicode[STIX]{x1D6E5},T)$ such that every panel of 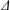 $\unicode[STIX]{x1D6E5}$ has at least
$\unicode[STIX]{x1D6E5}$ has at least  $k+1$ chambers. We show that a
$k+1$ chambers. We show that a 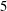 $5$-plump Tits triangle is parametrized and uniquely determined by a ring
$5$-plump Tits triangle is parametrized and uniquely determined by a ring 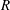 $R$ that is alternative and of stable rank
$R$ that is alternative and of stable rank  $2$. We use the connection between Tits triangles and the theory of Veldkamp planes as developed by Veldkamp and Faulkner to show existence.
$2$. We use the connection between Tits triangles and the theory of Veldkamp planes as developed by Veldkamp and Faulkner to show existence.
The work of the first author was partially supported by a grant from the DFG and the work of the second author was partially supported by a collaboration grant from the Simons Foundation.