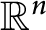Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Liu, Z.-S.
2023.
Spectrality of a class of Moran measures on the plane.
Acta Mathematica Hungarica,
Vol. 171,
Issue. 1,
p.
107.
Yang, M.-S.
and
Er, J.-W.
2025.
Spectrality of a class of self-affine measures with collinear digit sets on $$\mathbb{R}^{n}$$.
Analysis Mathematica,