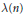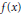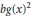Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Srinivasan, Anitha
2022.
Infinitely many sign changes of the Liouville function on 𝑋²+𝐷.
Proceedings of the American Mathematical Society,
Vol. 150,
Issue. 9,
p.
3799.
Klurman, Oleksiy
Shkredov, Ilya D.
and
Xu, Max Wenqiang
2023.
On the random Chowla conjecture.
Geometric and Functional Analysis,
Vol. 33,
Issue. 3,
p.
749.