Article contents
The Saddle-Point Method and the Li Coefficients
Published online by Cambridge University Press: 20 November 2018
Abstract
In this paper, we apply the saddle-point method in conjunction with the theory of the Nörlund–Rice integrals to derive precise asymptotic formula for the generalized Li coefficients established by Omar and Mazhouda. Actually, for any function  $F$ in the Selberg class
$F$ in the Selberg class 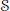 $\mathcal{S}$ and under the Generalized Riemann Hypothesis, we have
$\mathcal{S}$ and under the Generalized Riemann Hypothesis, we have
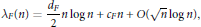 $${{\lambda }_{F}}(n)\,=\,\frac{{{d}_{F}}}{2}n\,\log \,n\,+\,{{c}_{F}}n\,+\,O(\sqrt{n}\,\log \,n),$$
$${{\lambda }_{F}}(n)\,=\,\frac{{{d}_{F}}}{2}n\,\log \,n\,+\,{{c}_{F}}n\,+\,O(\sqrt{n}\,\log \,n),$$
with
 $${{c}_{F}}\,=\,\frac{{{d}_{F}}}{2}(\gamma \,-\,1)\,+\,\frac{1}{2}\log (\lambda \text{Q}_{F}^{2}),\,\,\lambda \,=\,\prod\limits_{j=1}^{r}{\lambda _{j}^{2{{\lambda }_{j}}}},$$
$${{c}_{F}}\,=\,\frac{{{d}_{F}}}{2}(\gamma \,-\,1)\,+\,\frac{1}{2}\log (\lambda \text{Q}_{F}^{2}),\,\,\lambda \,=\,\prod\limits_{j=1}^{r}{\lambda _{j}^{2{{\lambda }_{j}}}},$$
where  $\gamma $ is the Euler's constant and the notation is as below.
$\gamma $ is the Euler's constant and the notation is as below.
Information
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 2011
References
- 4
- Cited by

