Article contents
Quantum Unique Ergodicity on Locally Symmetric Spaces: the Degenerate Lift
Published online by Cambridge University Press: 20 November 2018
Abstract
Given a measure  ${{\bar{\mu }}_{\infty }}$ on a locally symmetric space
${{\bar{\mu }}_{\infty }}$ on a locally symmetric space  $Y=\Gamma \backslash G/K$ obtained as a weak-
$Y=\Gamma \backslash G/K$ obtained as a weak-  $*$ limit of probability measures associated with eigenfunctions of the ring of invariant differential operators, we construct a measure
$*$ limit of probability measures associated with eigenfunctions of the ring of invariant differential operators, we construct a measure  ${{\bar{\mu }}_{\infty }}$ on the homogeneous space
${{\bar{\mu }}_{\infty }}$ on the homogeneous space 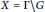 $X=\Gamma \backslash G$ that lifts
$X=\Gamma \backslash G$ that lifts  ${{\bar{\mu }}_{\infty }}$ and is invariant by a connected subgroup
${{\bar{\mu }}_{\infty }}$ and is invariant by a connected subgroup 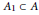 ${{A}_{1}}\subset A$ of positive dimension, where
${{A}_{1}}\subset A$ of positive dimension, where 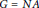 $G=NAK$ is an Iwasawa decomposition. If the functions are, in addition, eigenfunctions of the Hecke operators, then
$G=NAK$ is an Iwasawa decomposition. If the functions are, in addition, eigenfunctions of the Hecke operators, then  ${{\bar{\mu }}_{\infty }}$ is also the limit of measures associated with Hecke eigenfunctions on
${{\bar{\mu }}_{\infty }}$ is also the limit of measures associated with Hecke eigenfunctions on  $X$ . This generalizes results of the author with A. Venkatesh in the case where the spectral parameters stay away from the walls of the Weyl chamber.
$X$ . This generalizes results of the author with A. Venkatesh in the case where the spectral parameters stay away from the walls of the Weyl chamber.
Information
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 2015
References
- 3
- Cited by

