No CrossRef data available.
Article contents
Quadratic forms and Linkage of Quaternion Algebras
Published online by Cambridge University Press: 20 November 2018
Abstract
Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.
A field F satisfies n-linkage on a subset of Ḟ if whenever the quaternion algebras
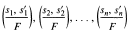
are equal in Br(F) there exist z ∈ Ḟ with
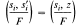
for i = 1, 2, . . .,n. This linkage of quaternion algebras is examined and its relationship to the torsion freeness of I2(F) and to the strong approximation property is investigated.
Information
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 1988
References
1.
Elman, R. and Lam, T. Y., Pfister forms and K-theory of fields, J. Algebra
23 (1972), pp. 181–213.Google Scholar
2.
Elman, R. and Lam, T. Y., Quadratic forms over formally real fields and Pythagorean fields, Amer. J. Math.
94 (1972), pp. 1155–1194.Google Scholar
3.
Elman, R. and Lam, T. Y., Quadratic forms and the u-invariant II, Invent. Math.
21 (1973), pp. 125–137.Google Scholar
4.
Elman, R. and Lam, T. Y., Classification theorems for quadratic forms over fields, Comment. Math. Helv.
49 (1974), pp. 373–381.Google Scholar
5.
Kaplansky, I., Frohlich's local quadratic forms, J. Reine Angew. Math.
239 (1969), pp. 74–77.Google Scholar
7.
Marshall, M., Abstract Witt rings, Queen papers in Pure and Applied Mathematics
57 (1980).Google Scholar

