Article contents
Products and Cardinal Invariants of Minimal Topological Groups
Published online by Cambridge University Press: 20 November 2018
Abstract
It is a question of Arhangel'skiĭ [1] (Problem 2) whether the identity ψ(G) = X(G) holds for every minimal Hausdorff topological group G = 〈G,u〉). (Here, as usual, ψ(G), the pseudocharacter of G, is the least cardinal number K for which there is 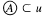 such that
such that 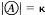 and
and 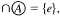 and x(G), the character of G,is the least cardinality of a local base at e for (〈G,u〉.) That 〈G, u〉 is minimal means that, if v is a Hausdorff topological group topology for G and v ⊂ u, then v = u.
and x(G), the character of G,is the least cardinality of a local base at e for (〈G,u〉.) That 〈G, u〉 is minimal means that, if v is a Hausdorff topological group topology for G and v ⊂ u, then v = u.
In this paper, we give some conditions on G sufficient to ensure a positive response to Arhangel'skiï's question, and we offer an example which responds negatively to a question on minimal groups posed some years ago (cf. [6] (p. 107) and [4] (p. 259)).
Information
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 1986
References
- 5
- Cited by

