Published online by Cambridge University Press: 20 November 2018
The characteristic polynomial 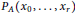 ${{P}_{A}}({{x}_{0}},...,{{x}_{r}})$ of an
${{P}_{A}}({{x}_{0}},...,{{x}_{r}})$ of an  $r$-tuple
$r$-tuple 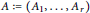 $A\,:=({{A}_{1}},...,{{A}_{r}})$ of
$A\,:=({{A}_{1}},...,{{A}_{r}})$ of 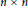 $n\times n$-matrices is defined as
$n\times n$-matrices is defined as
1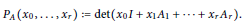 $${{P}_{A}}({{x}_{0}},...,{{x}_{r}}):=\det ({{x}_{0}}I+{{x}_{1}}{{A}_{1}}+\ldots +{{x}_{r}}{{A}_{r}}).$$
$${{P}_{A}}({{x}_{0}},...,{{x}_{r}}):=\det ({{x}_{0}}I+{{x}_{1}}{{A}_{1}}+\ldots +{{x}_{r}}{{A}_{r}}).$$
We show that if  $r\,\,3$ and
$r\,\,3$ and 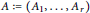 $A\,:=({{A}_{1}},...,{{A}_{r}})$ is an
$A\,:=({{A}_{1}},...,{{A}_{r}})$ is an  $r$-tuple of
$r$-tuple of 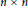 $n\times n$-matrices in general position, then up to conjugacy, there are only finitely many
$n\times n$-matrices in general position, then up to conjugacy, there are only finitely many  $r$-tuples
$r$-tuples  $A'\,:=(A_{1}^{'},...,A_{r}^{'})$ such that
$A'\,:=(A_{1}^{'},...,A_{r}^{'})$ such that 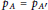 ${{p}_{A}}={{p}_{A'}}$. Equivalently, the locus of determinantal hypersurfaces of degree
${{p}_{A}}={{p}_{A'}}$. Equivalently, the locus of determinantal hypersurfaces of degree 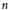 $n$ in
$n$ in 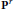 ${{\text{P}}^{r}}$ is irreducible of dimension
${{\text{P}}^{r}}$ is irreducible of dimension 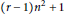 $(r-1){{n}^{2}}+1$.
$(r-1){{n}^{2}}+1$.