Published online by Cambridge University Press: 29 July 2020
For positive integers n and d > 0, let 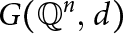 $G(\mathbb {Q}^n,\; d)$ denote the graph whose vertices are the set of rational points
$G(\mathbb {Q}^n,\; d)$ denote the graph whose vertices are the set of rational points 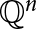 $\mathbb {Q}^n$, with
$\mathbb {Q}^n$, with 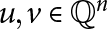 $u,v \in \mathbb {Q}^n$ being adjacent if and only if the Euclidean distance between u and v is equal to d. Such a graph is deemed “non-trivial” if d is actually realized as a distance between points of
$u,v \in \mathbb {Q}^n$ being adjacent if and only if the Euclidean distance between u and v is equal to d. Such a graph is deemed “non-trivial” if d is actually realized as a distance between points of 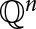 $\mathbb {Q}^n$. In this paper, we show that a space
$\mathbb {Q}^n$. In this paper, we show that a space 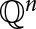 $\mathbb {Q}^n$ has the property that all pairs of non-trivial distance graphs
$\mathbb {Q}^n$ has the property that all pairs of non-trivial distance graphs 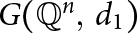 $G(\mathbb {Q}^n,\; d_1)$ and
$G(\mathbb {Q}^n,\; d_1)$ and 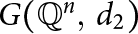 $G(\mathbb {Q}^n,\; d_2)$ are isomorphic if and only if n is equal to 1, 2, or a multiple of 4. Along the way, we make a number of observations concerning the clique number of
$G(\mathbb {Q}^n,\; d_2)$ are isomorphic if and only if n is equal to 1, 2, or a multiple of 4. Along the way, we make a number of observations concerning the clique number of 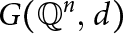 $G(\mathbb {Q}^n,\; d)$.
$G(\mathbb {Q}^n,\; d)$.