No CrossRef data available.
Article contents
On Basis Constants and Duality in Banach Spaces
Published online by Cambridge University Press: 20 November 2018
Abstract
Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.
Every Banach space with a non-shrinking (unconditional) basis (Xi ) can be renormed so that the biorthogonal sequence  has a much smaller (unconditional) basis constant than (xi ). On the other hand, if the unconditional constant of
has a much smaller (unconditional) basis constant than (xi ). On the other hand, if the unconditional constant of 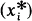 is C < 2 then the unconditional constant of (xi ) is at most C/(2—C). This estimate is sharp.
is C < 2 then the unconditional constant of (xi ) is at most C/(2—C). This estimate is sharp.
Information
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 1979
References
1.
Davis, W. J., Dean, D. W., Lin, B-L., Bibasic sequences and norming basic sequences,
Transactions AMS
176 (1973), 89-102.Google Scholar
2.
Davis, W. J., Johnson, W. B., A renaming of nonreflexive spaces,
Proc. AMS
37 (1973), 486-488.Google Scholar
3.
Lindenstrauss, J., Tzafriri, L., Classical Banach spaces I,
Springer-Verlag, Berlin
1977.Google Scholar
4.
Singer, I., On the constants of basic sequences in Banach spaces,
Studia Math.
31 (1968), 125-134.Google Scholar
5.
Singer, I., Bases in Banach spaces I,
Springer-Verlag, Berlin-Heidelberg, New York
1970.Google Scholar

