Hostname: page-component-cb9f654ff-h4f6x Total loading time: 0 Render date: 2025-08-30T22:06:43.076Z Has data issue: false hasContentIssue false
  • English
  • Français

A Non-Normal Function Whose Derivative is of Hardy Class Hp , 0<p<1

Published online by Cambridge University Press:  20 November 2018

Shinji Yamashita
Shinji Yamashita*
Affiliation:
Department of Mathematics, Tokyo Metropolitan University Fukazawa, Setagaya-Ku, Tokyo, 158 Japan
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

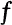Let Hp(0<p≤∞) be the Hardy class of functions holomorphic in D={|z|<1}, and let f be a function holomorphic in D. It follows from the well known theorem [1, Theorem 3.11, p. 42] that if (I) , then (II) f can be extended continuously to the closed disk {|z|≤1}, and consequently, (III) f is normal in D in the sense of O. Lehto and K. I. Virtanen [2]. Since there is a considerable gap between (II) and (III), one would suspect that the condition (I) might be much stronger than necessary to obtain the conclusion (III). However, it is curious that the implication (I)⇒(III) is sharp as the following theorem shows.

Information

Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 23 , Issue 4 , 01 December 1980 , pp. 499 - 500
Copyright
Copyright © Canadian Mathematical Society 1980

References

1. Duren, Peter L., Theory of Hp spaces. Academic Press, New York and London, 1970.Google Scholar
2. Lehto, Olli and Virtanen, Kaarlo I., Boundary behaviour and normal meromorphic functions. Acta Math. 97 (1957), 47-65.Google Scholar
3. Protas, David, Blaschke products with derivative in Hp and Bp. Michigan Math. J. 20 (1973).393-396.Google Scholar