Article contents
Non Cohen-Macaulay Vector Invariants and a Noether Bound for a Gorenstein Ring of Invariants
Published online by Cambridge University Press: 20 November 2018
Abstract
This paper contains two essentially independent results in the invariant theory of finite groups. First we prove that, for any faithful representation of a non-trivial  $p$ -group over a field of characteristic
$p$ -group over a field of characteristic  $p$ , the ring of vector invariants of
$p$ , the ring of vector invariants of 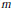 $m$ copies of that representation is not Cohen-Macaulay for
$m$ copies of that representation is not Cohen-Macaulay for 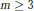 $m\,\ge \,3$ . In the second section of the paper we use Poincaré series methods to produce upper bounds for the degrees of the generators for the ring of invariants as long as that ring is Gorenstein. We prove that, for a finite non-trivial group
$m\,\ge \,3$ . In the second section of the paper we use Poincaré series methods to produce upper bounds for the degrees of the generators for the ring of invariants as long as that ring is Gorenstein. We prove that, for a finite non-trivial group 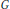 $G$ and a faithful representation of dimension
$G$ and a faithful representation of dimension  $n$ with
$n$ with 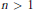 $n\,>\,1$ , if the ring of invariants is Gorenstein then the ring is generated in degrees less than or equal to
$n\,>\,1$ , if the ring of invariants is Gorenstein then the ring is generated in degrees less than or equal to 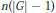 $n(\left| G \right|\,-\,1)$ . If the ring of invariants is a hypersurface, the upper bound can be improved to
$n(\left| G \right|\,-\,1)$ . If the ring of invariants is a hypersurface, the upper bound can be improved to 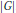 $\left| G \right|$ .
$\left| G \right|$ .
Keywords
Information
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 1999
References
- 13
- Cited by

