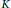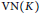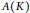Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
ESMAILVANDI, REZA
and
NEMATI, MEHDI
2021.
SOME HOMOLOGICAL PROPERTIES OF FOURIER ALGEBRAS ON HOMOGENEOUS SPACES.
Bulletin of the Australian Mathematical Society,
Vol. 104,
Issue. 1,
p.
132.
ESMAILVANDI, REZA
NEMATI, MEHDI
and
KUMAR, NAGESWARAN SHRAVAN
2023.
ON THE ALGEBRAS AND OF AN ULTRASPHERICAL HYPERGROUP .
Journal of the Australian Mathematical Society,
Vol. 115,
Issue. 3,
p.
337.