No CrossRef data available.
Published online by Cambridge University Press: 20 November 2018
This paper is concerned with the structure of inner 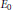 ${{E}_{0}}$-semigroups. We show that any inner
${{E}_{0}}$-semigroups. We show that any inner 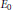 ${{E}_{0}}$-semigroup acting on an infinite factor
${{E}_{0}}$-semigroup acting on an infinite factor 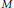 $M$ is completely determined by a continuous tensor product system of Hilbert spaces in
$M$ is completely determined by a continuous tensor product system of Hilbert spaces in 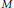 $M$ and that the product system associated with an inner
$M$ and that the product system associated with an inner 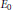 ${{E}_{0}}$-semigroup is a complete cocycle conjugacy invariant.
${{E}_{0}}$-semigroup is a complete cocycle conjugacy invariant.