Published online by Cambridge University Press: 20 November 2018
Following a similar result of Uspenskij on the unitary group of a separable Hilbert space, we show that, with respect to the lower (or Roelcke) uniform structure, the Polish group  $G\,=\,\text{Aut(}\mu \text{)}$ of automorphisms of an atomless standard Borel probability space
$G\,=\,\text{Aut(}\mu \text{)}$ of automorphisms of an atomless standard Borel probability space  $(X,\,\mu )$ is precompact. We identify the corresponding compactification as the space of Markov operators on
$(X,\,\mu )$ is precompact. We identify the corresponding compactification as the space of Markov operators on  ${{L}_{2}}(\mu )$ and deduce that the algebra of right and left uniformly continuous functions, the algebra of weakly almost periodic functions, and the algebra of Hilbert functions on
${{L}_{2}}(\mu )$ and deduce that the algebra of right and left uniformly continuous functions, the algebra of weakly almost periodic functions, and the algebra of Hilbert functions on 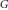 $G$, i.e., functions on
$G$, i.e., functions on 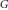 $G$ arising from unitary representations, all coincide. Again following Uspenskij, we also conclude that
$G$ arising from unitary representations, all coincide. Again following Uspenskij, we also conclude that 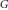 $G$ is totally minimal.
$G$ is totally minimal.