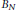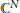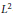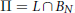Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Kot, Piotr
2007.
p-Radial Exceptional Sets and Conformal Mappings.
Canadian Mathematical Bulletin,
Vol. 50,
Issue. 4,
p.
579.
Kot, Piotr
2007.
Homogeneous polynomials on strictly convex domains.
Proceedings of the American Mathematical Society,
Vol. 135,
Issue. 12,
p.
3895.
Kot, Piotr
2007.
Boundary functions in L 2 H $$(\mathbb{B}^n )$$.
Czechoslovak Mathematical Journal,
Vol. 57,
Issue. 1,
p.
29.
Kot, Piotr
2009.
Boundary functions on a bounded balanced domain.
Czechoslovak Mathematical Journal,
Vol. 59,
Issue. 2,
p.
371.