Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Zamani, Ali
and
Moslehian, Mohammad Sal
2016.
Norm-parallelism in the geometry of Hilbert C∗-modules.
Indagationes Mathematicae,
Vol. 27,
Issue. 1,
p.
266.
Zamani, Ali
2016.
The operator-valued parallelism.
Linear Algebra and its Applications,
Vol. 505,
Issue. ,
p.
282.
Grover, Priyanka
2017.
Orthogonality of matrices in the Ky Fan k-norms.
Linear and Multilinear Algebra,
Vol. 65,
Issue. 3,
p.
496.
Wójcik, Paweł
2017.
Norm-parallelism in classical M-ideals.
Indagationes Mathematicae,
Vol. 28,
Issue. 2,
p.
287.
Sal Moslehian, Mohammad
and
Zamani, Ali
2017.
Characterizations of Operator Birkhoff–James Orthogonality.
Canadian Mathematical Bulletin,
Vol. 60,
Issue. 4,
p.
816.
Gohari, M. Mohammadi
and
Amyari, M.
2019.
Parallelism in Hilbert $K(\mathcal{H})$-modules.
Tbilisi Mathematical Journal,
Vol. 12,
Issue. 2,
BOTTAZZI, T.
CONDE, C.
MOSLEHIAN, M. S.
WÓJCIK, P.
and
ZAMANI, A.
2019.
ORTHOGONALITY AND PARALLELISM OF OPERATORS ON VARIOUS BANACH SPACES.
Journal of the Australian Mathematical Society,
Vol. 106,
Issue. 2,
p.
160.
Zamani, Ali
2019.
Characterization of numerical radius parallelism in $$C^*$$C∗-algebras.
Positivity,
Vol. 23,
Issue. 2,
p.
397.
Zamani, Ali
2019.
Characterizations of Norm–Parallelism in Spaces of Continuous Functions.
Bulletin of the Iranian Mathematical Society,
Vol. 45,
Issue. 2,
p.
557.
Zamani, Ali
Moslehian, Mohammad Sal
Chien, Mao-Ting
and
Nakazato, Hiroshi
2019.
Norm-parallelism and the Davis–Wielandt radius of Hilbert space operators.
Linear and Multilinear Algebra,
Vol. 67,
Issue. 11,
p.
2147.
Feki, Kais
and
Ahmed Mahmoud, Sid Ahmed Ould
2020.
Davis–Wielandt shells of semi-Hilbertian space operators and its applications.
Banach Journal of Mathematical Analysis,
Vol. 14,
Issue. 3,
p.
1281.
Mohammadi Gohari, M.
and
Amyari, M.
2020.
The Operator-Valued Parallelism and Norm-Parallelism in Matrices.
Indian Journal of Pure and Applied Mathematics,
Vol. 51,
Issue. 4,
p.
1305.
Mehrazin, Marzieh
Amyari, Maryam
and
Zamani, Ali
2020.
Numerical Radius Parallelism of Hilbert Space Operators.
Bulletin of the Iranian Mathematical Society,
Vol. 46,
Issue. 3,
p.
821.
Bottazzi, Tamara
Conde, Cristian
and
Feki, Kais
2021.
On A-Parallelism and A-Birkhoff–James Orthogonality of Operators.
Results in Mathematics,
Vol. 76,
Issue. 4,
Grover, Priyanka
and
Singla, Sushil
2021.
Operator Theory, Functional Analysis and Applications.
Vol. 282,
Issue. ,
p.
293.
Mabrouk, Mohamed
and
Noor, Samah
2022.
On the joint numerical radius parallelism of operators.
Advances in Operator Theory,
Vol. 7,
Issue. 2,
Amyari, M.
Torabian, M.
and
Moradian Khibary, M.
2023.
Approximate isosceles $$\omega $$-orthogonality and approximate $$\omega $$-parallelism.
Boletín de la Sociedad Matemática Mexicana,
Vol. 29,
Issue. 3,
Alomari, M. W.
2023.
On the Davis–Wielandt radius inequalities of Hilbert space operators.
Linear and Multilinear Algebra,
Vol. 71,
Issue. 11,
p.
1804.
Wójcik, Paweł
and
Zamani, Ali
2023.
From norm derivatives to orthogonalities in Hilbert C*-modules.
Linear and Multilinear Algebra,
Vol. 71,
Issue. 6,
p.
875.
Bi, Jiaye
Xie, Huayou
and
Li, Yongjin
2024.
On a parallelism related to the g-angle.
Filomat,
Vol. 38,
Issue. 32,
p.
11227.
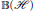 $\mathbb{B}\left( H \right)$ of bounded linear operators acting on a Hilbert space
$\mathbb{B}\left( H \right)$ of bounded linear operators acting on a Hilbert space  $H$ . Among other things, we investigate the relationship between the approximate parallelism and norm of inner derivations on
$H$ . Among other things, we investigate the relationship between the approximate parallelism and norm of inner derivations on 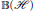 $\mathbb{B}\left( H \right)$ . We also characterize the parallel elements of a
$\mathbb{B}\left( H \right)$ . We also characterize the parallel elements of a 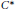 ${{C}^{*}}$ -algebra by using states. Finally we utilize the linking algebra to give some equivalent assertions regarding parallel elements in a Hilbert
${{C}^{*}}$ -algebra by using states. Finally we utilize the linking algebra to give some equivalent assertions regarding parallel elements in a Hilbert 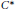 ${{C}^{*}}$ -module.
${{C}^{*}}$ -module.
