Published online by Cambridge University Press: 20 November 2018
In 2012, Gubeladze (Adv. Math. 2012) introduced the notion of  $k$-convex-normal polytopes to show that integral polytopes all of whose edges are longer than
$k$-convex-normal polytopes to show that integral polytopes all of whose edges are longer than 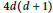 $4d(d+1)$ have the integer decomposition property. In the first part of this paper we show that for lattice polytopes there is no diòerence between
$4d(d+1)$ have the integer decomposition property. In the first part of this paper we show that for lattice polytopes there is no diòerence between  $k$- and
$k$- and 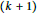 $(k+1)$-convex-normality (for
$(k+1)$-convex-normality (for 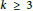 $k\ge 3$) and improve the bound to
$k\ge 3$) and improve the bound to 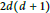 $2d(d+1)$. In the second part we extend the definition to pairs of polytopes. Given two rational polytopes
$2d(d+1)$. In the second part we extend the definition to pairs of polytopes. Given two rational polytopes  $P$ and
$P$ and 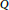 $\text{Q}$, where the normal fan of
$\text{Q}$, where the normal fan of  $P$ is a refinement of the normal fan of
$P$ is a refinement of the normal fan of 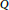 $\text{Q}$, if every edge
$\text{Q}$, if every edge 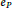 ${{e}_{P}}$ of
${{e}_{P}}$ of  $P$ is at least
$P$ is at least  $d$ times as long as the corresponding face (edge or vertex)
$d$ times as long as the corresponding face (edge or vertex) 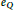 ${{e}_{\text{Q}}}$ of
${{e}_{\text{Q}}}$ of 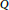 $\text{Q}$, then
$\text{Q}$, then 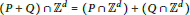 $(P+\text{Q})\cap {{\mathbb{Z}}^{d}}=(P\cap {{\mathbb{Z}}^{d}})+(\text{Q}\cap {{\mathbb{Z}}^{d}})$.
$(P+\text{Q})\cap {{\mathbb{Z}}^{d}}=(P\cap {{\mathbb{Z}}^{d}})+(\text{Q}\cap {{\mathbb{Z}}^{d}})$.