No CrossRef data available.
Article contents
Convergence of Averaged Occupation Times
Published online by Cambridge University Press: 20 November 2018
Extract
Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.
Let X = {Xt, t ≥ 0} be a stationary Markov process with values in a measurable space (S, ℬ), transition function p, and initial distribution concentrated at a point x ∊ S. The occupation times of a set A ∊ ℬ are defined for t ≥ 0 by
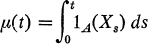
where 1A is the indicator function of A. The expected occupation times are given by
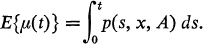
Information
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 1975
References
2.
Brosamler, G. A., The asymptotic behavior of certain additive junctionals of Brownian motion, Inventiones Math., 20 (1973), 87-96.Google Scholar
3.
Chung, K. L. and Erdös, P., Probability limit theorems assuming only the first moment I, Memoirs of the Amer. Math. Sac., 6 (1951).Google Scholar
4.
Darling, D. A. and Kac, M., On occupation times for Markov processes, Trans. Amer. Math. Soc.
84 (1957), 444-458.Google Scholar
5.
Feller, W., An introduction to probability theory and its applications, Volume II (Wiley, New York, 1971).Google Scholar
6.
Kallianpur, G. and Robbins, H., Ergodic property of the Brownian motion process, Proc. Nat. Acad. Sci. U.S.A., 39 (1953), 525-533.Google Scholar

