No CrossRef data available.
Article contents
Complexifying Lie Group Actions on Homogeneous Manifolds of Non-compact Dimension Two
Published online by Cambridge University Press: 20 November 2018
Abstract
Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.
If 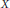 $X$ is a connected complex manifold with
$X$ is a connected complex manifold with 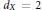 ${{d}_{X}}\,=\,2$ that admits a (connected) Lie group
${{d}_{X}}\,=\,2$ that admits a (connected) Lie group 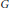 $G$ acting transitively as a group of holomorphic transformations, then the action extends to an action of the complexification
$G$ acting transitively as a group of holomorphic transformations, then the action extends to an action of the complexification 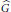 $\widehat{G}$ of
$\widehat{G}$ of 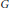 $G$ on
$G$ on 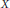 $X$ except when either the unit disk in the complex plane or a strictly pseudoconcave homogeneous complex manifold is the base or fiber of some homogeneous fibration of
$X$ except when either the unit disk in the complex plane or a strictly pseudoconcave homogeneous complex manifold is the base or fiber of some homogeneous fibration of 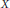 $X$ .
$X$ .
Information
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 2014
References
[Abe76]
Abels, H., Proper transformation groups. In: Transformation groups. (Proc. Conf., Univ. Newcastle upon Tyne, Newcastle upon Tyne, 1976), London Math. Soc. Lecture Note Series, 26, Cambridge University Press, Cambridge, 1977, pp. 237–248.Google Scholar
[Abe82]
Abels, H.,Some topological aspects of proper group actions; noncompact dimension of groups.
J. London Math. Soc. (2)
25 (1982), no. 3, 525–538. http://dx.doi.org/10.1112/jlms/s2-25.3.525
Google Scholar
[Akh77]
Ahiezer, D. N., Dense orbits with two endpoints.
(Russian) Izv. Akad. Nauk SSSR Ser. Mat.
41 (1977), no. 2, 308–324, 477; Math. USSR-Izv. 11 (1977), no. 2, 293–307.Google Scholar
[Akh79]
Ahiezer, D. N., Algebraic groups that are transitive in the complement to a homogeneous hypersurface. (Russian) Dokl. Akad. Nauk SSSR 245 (1979), no. 2, 281–284; Soviet Math. Dokl. 20 (1979), no. 2, 278–281.Google Scholar
[Akh83]
Ahiezer, D. N., Complex n-dimensional homogeneous spaces homotopically equivalent to (2n-2)-dimensional compact manifolds. Selecta Math. Soviet
3(1983/84), no. 3, 286–290.Google Scholar
[Akh13]
Ahiezer, D. N., Real group orbits on flag manifolds. In: Lie groups: structure, actions, and representations Progress in Mathematics, 306, Birkhäuser Basel, 2013, pp. 1–24.Google Scholar
[AG94]
Akhiezer, D. and Gilligan, B., On complex homogeneous spaces with top homology in codimension two.Canad. J. Math.
46 (1994), no. 5, 897–919. http://dx.doi.org/10.4153/CJM-1994-051-x
Google Scholar
[BoMo47]
Bochner, S. and Montgomery, D., Groups on analytic manifolds. Ann. of Math.
48 (1947), 659–669. http://dx.doi.org/10.2307/1969133
Google Scholar
[Bor53]
Borel, A., Les bouts des espaces homogánes de groupes de Lie.
Ann. of Math. (2)
58 (1953), 443–457. http://dx.doi.org/10.2307/1969747
Google Scholar
[Che51]
Chevalley, C., Théorie des groupes de Lie. Tome II. Groupes algébriques. Actualités Sci. Ind., 1152, Hermann &Cie., Paris, 1951.Google Scholar
[FHW]
Fels, G., Huckleberry, A. T., and J. A.Wolf, Cycle spaces of flag domains. A complex geometric viewpoint. Progress in Mathematics, 245, Birkhäuser Boston, Inc., Boston, MA, 2006.Google Scholar
[Gil91]
Gilligan, B., On the ends of complex manifolds homogeneous under a Lie group. In: Several complex variables and complex geometry, Part 2 (Santa Cruz, CA, 1989), Proc. Sympos. Pure Math., 52, American Mathematical Society, Providence, RI, 1991, pp. 217–224.Google Scholar
[Gil95]
Gilligan, B., Complex homogeneous spaces of real groups with top homology in codimension two.Ann. Global Anal. Geom.
13 (1995), no. 3, 303–314. http://dx.doi.org/10.1007/BF00773662
Google Scholar
[GH98]
Gilligan, B. and Heinzner, P., Globalization of holomorphic actions on principal bundles.Math. Nachr.
189 (1998), 145–156. http://dx.doi.org/10.1002/mana.19981890109
Google Scholar
[GH09]
Gilligan, B. and Huckleberry, A. T., Fibrations and globalizations of compact homogeneous CR-Manifolds.(Russian) Izv. Ross. Akad. Nauk Ser. Mat. 73 (2009), no. 3, 67–126; translation in: Izv. Math. 73 (2009), no. 3, 501–553. http://dx.doi.org/10.4213/im2734
Google Scholar
[Hir70]
Hironaka, H., Desingularizations of complex-analytic varieties. (French) In: Actes du Congrás International des Mathématiciens (Nice, 1970), Tome 2, Gauthier-Villars, Paris, 1971, pp. 627–631.Google Scholar
[Hoch65]
Hochschild, G., The structure of Lie groups.
Holden-Day Inc., San Franscisco-London-Amsterdam, 1965.Google Scholar
[HO81]
Huckleberry, A. T. and Oeljeklaus, E., Homogeneous spaces from a complex analytic viewpoint. In: Manifolds and Lie groups. (Notre Dame, Ind., 1980) Progress in Math., 14, Birkhäuser, Boston, MA, 1981, pp. 159–186.Google Scholar
[HO84]
Huckleberry, A. T. and Oeljeklaus, E., Classification theorems for almost homogeneous spaces. Institut E´ lie Cartan, 9, Universite´ de Nancy, Institut E´ lie Cartan, Nancy, 1984.Google Scholar
[HS81]
Huckleberry, A. T. and Oeljeklaus, E.
Huckleberry, A. T. and Snow, D., A classification of strictly pseudoconcave homogeneous manifolds.Ann. Scuola Norm. Sup. Pisa
8 (1981), no. 2, 231–255.Google Scholar
[HS82]
Huckleberry, A. T. and Oeljeklaus, E.
Huckleberry, A. T. and Snow, D., Almost-homogeneous Kähler manifolds with hypersurface orbits.Osaka J. Math.
19 (1982), no. 4, 763–786.Google Scholar
[Ka67]
Kaup, W., Reelle Transformationsgruppen und invariante Metriken auf komplexen Räumen.Invent. Math.
3 (1967), 43–70. http://dx.doi.org/10.1007/BF01425490
Google Scholar
[Mal75]
Malyshev, F. M., Complex homogeneous spaces of semisimple Lie groups of the first category. (Russian) Izv. Akad. Nauk SSSR, Ser. Mat.
39 (1975),no. 5, 992–1002, 1219; translation in: Math. USSR Izv. 9 (1977), no. 5, 939–949.Google Scholar
[Mal77]
Malyshev, F. M., Complex homogeneous spaces of semisimple Lie groups of type Dn.
(Russian) Izv. Akad. Nauk SSSR, Ser. Mat.
41 (1977), no. 4, 829–852, 959; translation in: Math. USSR Izv. 11 (1977), no. 4, 783–805.Google Scholar
[Mat79]
Matsuki, T., The orbits of affine symmetric spaces under the action of minimal parabolic subgroups.
J. Math. Soc. Japan
31 (1979), no. 2, 331–357. http://dx.doi.org/10.2969/jmsj/03120331
Google Scholar
[Mat82]
Matsuki, T., Orbits on affine symmetric spaces under the action of parabolic subgroups. Hiroshima Math. J. 12 (1982), no. 2, 307–320.Google Scholar
[Mat60]
Matsushima, Y., Espaces homogánes de Stein des groupes de Lie complexes.Nagoya Math. J.
16 (1960), 205–218.Google Scholar
[MN63]
Morimoto, A. and Nagano, T.,On pseudo-conformal transformations of hypersurfaces.J. Math. Soc. Japan.
15 (1963), 289–300. http://dx.doi.org/10.2969/jmsj/01530289
Google Scholar
[Mos55]
Mostow, G. D., On covariant fiberings of Klein spaces. Amer. J. Math.
77 (1955), 247–278. http://dx.doi.org/10.2307/2372530
Google Scholar
[Mos62]
Mostow, G. D., Covariant fiberings of Klein spaces. II.
Amer. J. Math.
84 (1962), 466–474. http://dx.doi.org/10.2307/2372983
Google Scholar
[OR84]
Oeljeklaus, K. andRichthofer, W., Homogeneous complex surfaces.
Math. Ann.
268 (1984), no. 3, 273–292. http://dx.doi.org/10.1007/BF01457059
Google Scholar
[Oni60]
Onishchik, A. L., Complex hulls of compact homogeneous spaces. (Russian) Dokl. Akad. Nauk SSSR 130 (1960), 726–729; translation in: Soviet Math. Dokl.
1 (1960) 88–91.Google Scholar
[Oni62]
Onishchik, A. L., Inclusion relations among transitive compact transformation groups.
(Russian) Trudy Mosk. Mat. Obsc.
11 (1962), 199–242; translation in: Amer. Math. Soc. Transl. 50 (1966), 5–58.Google Scholar
[Oni69]
Onishchik, A. L., Decompositions of reductive Lie groups. (Russian) Mat. Sb. 80 (122) (1969), 553–599; translation in: Math. USSR Sb. 9 (1969), 515–554.Google Scholar
[Ste82]
Steinsiek, M., Transformation groups on homogeneous-rational manifolds.
Math. Ann.
260 (1982), no. 4, 423–435. http://dx.doi.org/10.1007/BF01457022
Google Scholar
[Wan52]
Wang, H.-C, Two-point homogeneous spaces.Ann. of Math. (2)
55 (1952), 177–191. http://dx.doi.org/10.2307/1969427
Google Scholar
[Weis66]
Weisfeiler, B., On one class of unipotent subgroups of semisimple algebraic groups.
arxiv:math/0005149v1; translated from Russian: Uspehi Mat. Nauk
21 (1966), 222–223.Google Scholar
[Wol69]
Wolf, J. A., The action of a real semisimple group on a complex flag manifold. I. Orbit structure and holomorphic arc components.
Bull. Amer. Math. Soc.
75 (1969), 1121–1237. http://dx.doi.org/10.1090/S0002-9904-1969-12359-1
Google Scholar

