No CrossRef data available.
Article contents
Certain nth Order Differential Inequalities in the Complex Plane
Published online by Cambridge University Press: 20 November 2018
Abstract
Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.
Let w(z) be regular in the unit disc U:|z|<l, with w(0) = 0 and let h(r, s, t) be a complex function defined in a domain D of C3. The author determines conditions on h such that if
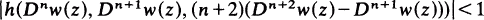
z∈U, then |w(z)|< 1 for z ∈ U and n= 0, 1, 2, …. Here Dnw(z) = (z/(l-z)n+1*w(z), where * stands for the Hadamard product (convolution). Some applications of the results to certain differential equations are given.
Information
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 1978
References
1.
Jack, I. S., Functions starlike and convex of order a,
J. London Math. Soc. (2)
3 (1971), 469-474.Google Scholar
2.
Miller, S. S., A class of differential inequalities implying boundedness,
III. J. of Math.
20 (1976), 647-649.Google Scholar
3.
Miller, S. S. and Mocanu, P. T., Second order differential inequalities in the complex plane, to appear.Google Scholar
4.
Ruscheweyh, S., New criteria for univalent functions,
Proc. Amer. Math. Soc. vol.
49 (1975), 109-115.Google Scholar

