Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Czaja, Wojciech
and
Powell, Alexander M.
2006.
Harmonic Analysis and Applications.
p.
79.
Alvarez, Josefina
Eydenberg, Michael S.
and
Obiedat, Hamed
2008.
The action of operator semigroups on the topological dual of the Beurling–Björck space.
Journal of Mathematical Analysis and Applications,
Vol. 339,
Issue. 1,
p.
405.
Grip, Niklas
and
Pfander, Götz E.
2008.
A discrete model for the efficient analysis of time-varying narrowband communication channels.
Multidimensional Systems and Signal Processing,
Vol. 19,
Issue. 1,
p.
3.
Chen, Weiqiang
and
Goh, Say Song
2010.
Band-limited refinable functions for wavelets and framelets.
Applied and Computational Harmonic Analysis,
Vol. 28,
Issue. 3,
p.
338.
Moritoh, Shinya
and
Tomoeda, Kyoko
2010.
A Further Decay Estimate for the Dziubański–Hernández Wavelets.
Canadian Mathematical Bulletin,
Vol. 53,
Issue. 1,
p.
133.
Milićević, Djordje
2010.
Large values of eigenfunctions on arithmetic hyperbolic surfaces.
Duke Mathematical Journal,
Vol. 155,
Issue. 2,
Ivanov, Kamen
Petrushev, Pencho
and
Xu, Yuan
2010.
Sub-exponentially localized kernels and frames induced by orthogonal expansions.
Mathematische Zeitschrift,
Vol. 264,
Issue. 2,
p.
361.
Bachmann, Sven
Michalakis, Spyridon
Nachtergaele, Bruno
and
Sims, Robert
2012.
Automorphic Equivalence within Gapped Phases of Quantum Lattice Systems.
Communications in Mathematical Physics,
Vol. 309,
Issue. 3,
p.
835.
Hou, Likun
and
Ji, Hui
2013.
Band-limited Wavelets and Framelets in Low Dimensions.
Journal of Fourier Analysis and Applications,
Vol. 19,
Issue. 4,
p.
731.
Pfander, Götz E.
2013.
Sampling of Operators.
Journal of Fourier Analysis and Applications,
Vol. 19,
Issue. 3,
p.
612.
Fukuda, Naohiro
Kinoshita, Tamotu
and
Uehara, Ion
2014.
On the wavelets having Gevrey regularities and subexponential decays.
Mathematische Nachrichten,
Vol. 287,
Issue. 5-6,
p.
546.
Gun, Chiou-Yueh
Wang, Kai-Cheng
Yang, Chi-I
and
Chang, Kuei-Fang
2015.
Pre-wavelet bases in Lebesgue spaces.
Journal of Inequalities and Applications,
Vol. 2015,
Issue. 1,
Pilipović, Stevan
Rakić, Dušan
Teofanov, Nenad
and
Vindas, Jasson
2016.
The wavelet transforms in Gelfand–Shilov spaces.
Collectanea Mathematica,
Vol. 67,
Issue. 3,
p.
443.
Mariën, Michaël
Audenaert, Koenraad M. R.
Van Acoleyen, Karel
and
Verstraete, Frank
2016.
Entanglement Rates and the Stability of the Area Law for the Entanglement Entropy.
Communications in Mathematical Physics,
Vol. 346,
Issue. 1,
p.
35.
Pathak, R. S.
and
Singh, Abhishek
2019.
Paley–Wiener–Schwartz type theorem for the wavelet transform.
Applicable Analysis,
Vol. 98,
Issue. 7,
p.
1324.
Pilipović, Stevan
Rakić, Dušan
Teofanov, Nenad
and
Vindas, Jasson
2020.
Multiresolution expansions and wavelets in Gelfand–Shilov spaces.
Revista de la Real Academia de Ciencias Exactas, Físicas y Naturales. Serie A. Matemáticas,
Vol. 114,
Issue. 2,
Wang, Kai-Cheng
2021.
A Jackson-type inequality associated with wavelet bases decomposition.
Journal of Inequalities and Applications,
Vol. 2021,
Issue. 1,
Singh, Abhishek
Raghuthaman, Nikhila
and
Rawat, Aparna
2021.
Paley–Wiener–Schwartz Type Theorem for Ultradistributional Wavelet Transform.
Complex Analysis and Operator Theory,
Vol. 15,
Issue. 4,
Xu, Yuan
2021.
Approximation and localized polynomial frame on conic domains.
Journal of Functional Analysis,
Vol. 281,
Issue. 12,
p.
109257.
Singh, Abhishek
and
Raghuthaman, Nikhila
2022.
Generalization of Paley–Wiener–Schwartz Type Theorem.
National Academy Science Letters,
Vol. 45,
Issue. 4,
p.
335.
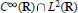 ${{C}^{\infty }}\,(\text{R})\,\cap \,{{L}^{2}}\,(R)$ . This is also true for wavelets with exponential decay. We show that one can construct wavelets in the class
${{C}^{\infty }}\,(\text{R})\,\cap \,{{L}^{2}}\,(R)$ . This is also true for wavelets with exponential decay. We show that one can construct wavelets in the class 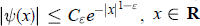 ${{C}^{\infty }}\,(\text{R})\,\cap \,{{L}^{2}}\,(R)$ that are “almost” of exponential decay and, moreover, they are band-limited. We do this by showing that we can adapt the construction of the Lemarié-Meyer wavelets
${{C}^{\infty }}\,(\text{R})\,\cap \,{{L}^{2}}\,(R)$ that are “almost” of exponential decay and, moreover, they are band-limited. We do this by showing that we can adapt the construction of the Lemarié-Meyer wavelets  $[\text{LM }\!\!]\!\!\text{ }$ that is found in
$[\text{LM }\!\!]\!\!\text{ }$ that is found in 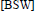 $[\text{BSW}]$ so that we obtain band-limited,
$[\text{BSW}]$ so that we obtain band-limited,  ${{C}^{\infty }}$ -wavelets on
${{C}^{\infty }}$ -wavelets on 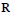 $R$ that have subexponential decay, that is, for every
$R$ that have subexponential decay, that is, for every 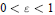 $0<\varepsilon <1$ , there exits
$0<\varepsilon <1$ , there exits  ${{C}_{\in }}\,>\,0$ such that
${{C}_{\in }}\,>\,0$ such that 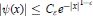 $|\psi (x)|\le {{C}_{\varepsilon }}{{e}^{-|x{{|}^{1-\varepsilon }}}}$ ,
$|\psi (x)|\le {{C}_{\varepsilon }}{{e}^{-|x{{|}^{1-\varepsilon }}}}$ , 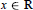 $x\in \text{R}$ . Moreover, all of its derivatives have also subexponential decay. The proof is constructive and uses the Gevrey classes of functions.
$x\in \text{R}$ . Moreover, all of its derivatives have also subexponential decay. The proof is constructive and uses the Gevrey classes of functions.
